5 Advantages and Disadvantages of Problem-Based Learning [+ Activity Design Steps]
Written by Marcus Guido
- Teaching Strategies

- Advantages of Problem-Based Learning
- Disadvantages of Problem-Based Learning
- Steps to Designing Problem-Based Learning Activities
Used since the 1960s, many teachers express concerns about the effectiveness of problem-based learning (PBL) in certain classroom settings.
Whether you introduce the student-centred pedagogy as a one-time activity or mainstay exercise, grouping students together to solve open-ended problems can present pros and cons.
Below are five advantages and disadvantages of problem-based learning to help you determine if it can work in your classroom.
If you decide to introduce an activity, there are also design creation steps and a downloadable guide to keep at your desk for easy reference.

1. Development of Long-Term Knowledge Retention
Students who participate in problem-based learning activities can improve their abilities to retain and recall information, according to a literature review of studies about the pedagogy .
The literature review states “elaboration of knowledge at the time of learning” -- by sharing facts and ideas through discussion and answering questions -- “enhances subsequent retrieval.” This form of elaborating reinforces understanding of subject matter , making it easier to remember.
Small-group discussion can be especially beneficial -- ideally, each student will get chances to participate.
But regardless of group size, problem-based learning promotes long-term knowledge retention by encouraging students to discuss -- and answer questions about -- new concepts as they’re learning them.
2. Use of Diverse Instruction Types

You can use problem-based learning activities to the meet the diverse learning needs and styles of your students, effectively engaging a diverse classroom in the process. In general, grouping students together for problem-based learning will allow them to:
- Address real-life issues that require real-life solutions, appealing to students who struggle to grasp abstract concepts
- Participate in small-group and large-group learning, helping students who don’t excel during solo work grasp new material
- Talk about their ideas and challenge each other in a constructive manner, giving participatory learners an avenue to excel
- Tackle a problem using a range of content you provide -- such as videos, audio recordings, news articles and other applicable material -- allowing the lesson to appeal to distinct learning styles
Since running a problem-based learning scenario will give you a way to use these differentiated instruction approaches , it can be especially worthwhile if your students don’t have similar learning preferences.
3. Continuous Engagement

Providing a problem-based learning challenge can engage students by acting as a break from normal lessons and common exercises.
It’s not hard to see the potential for engagement, as kids collaborate to solve real-world problems that directly affect or heavily interest them.
Although conducted with post-secondary students, a study published by the Association for the Study of Medical Education reported increased student attendance to -- and better attitudes towards -- courses that feature problem-based learning.
These activities may lose some inherent engagement if you repeat them too often, but can certainly inject excitement into class.
4. Development of Transferable Skills
Problem-based learning can help students develop skills they can transfer to real-world scenarios, according to a 2015 book that outlines theories and characteristics of the pedagogy .
The tangible contexts and consequences presented in a problem-based learning activity “allow learning to become more profound and durable.” As you present lessons through these real-life scenarios, students should be able to apply learnings if they eventually face similar issues.
For example, if they work together to address a dispute within the school, they may develop lifelong skills related to negotiation and communicating their thoughts with others.
As long as the problem’s context applies to out-of-class scenarios, students should be able to build skills they can use again.
5. Improvement of Teamwork and Interpersonal Skills

Successful completion of a problem-based learning challenge hinges on interaction and communication, meaning students should also build transferable skills based on teamwork and collaboration . Instead of memorizing facts, they get chances to present their ideas to a group, defending and revising them when needed.
What’s more, this should help them understand a group dynamic. Depending on a given student, this can involve developing listening skills and a sense of responsibility when completing one’s tasks. Such skills and knowledge should serve your students well when they enter higher education levels and, eventually, the working world.
1. Potentially Poorer Performance on Tests

Devoting too much time to problem-based learning can cause issues when students take standardized tests, as they may not have the breadth of knowledge needed to achieve high scores. Whereas problem-based learners develop skills related to collaboration and justifying their reasoning, many tests reward fact-based learning with multiple choice and short answer questions. Despite offering many advantages, you could spot this problem develop if you run problem-based learning activities too regularly.
2. Student Unpreparedness

Problem-based learning exercises can engage many of your kids, but others may feel disengaged as a result of not being ready to handle this type of exercise for a number of reasons. On a class-by-class and activity-by-activity basis, participation may be hindered due to:
- Immaturity -- Some students may not display enough maturity to effectively work in a group, not fulfilling expectations and distracting other students.
- Unfamiliarity -- Some kids may struggle to grasp the concept of an open problem, since they can’t rely on you for answers.
- Lack of Prerequisite Knowledge -- Although the activity should address a relevant and tangible problem, students may require new or abstract information to create an effective solution.
You can partially mitigate these issues by actively monitoring the classroom and distributing helpful resources, such as guiding questions and articles to read. This should keep students focused and help them overcome knowledge gaps. But if you foresee facing these challenges too frequently, you may decide to avoid or seldom introduce problem-based learning exercises.
3. Teacher Unpreparedness
If supervising a problem-based learning activity is a new experience, you may have to prepare to adjust some teaching habits . For example, overtly correcting students who make flawed assumptions or statements can prevent them from thinking through difficult concepts and questions. Similarly, you shouldn’t teach to promote the fast recall of facts. Instead, you should concentrate on:
- Giving hints to help fix improper reasoning
- Questioning student logic and ideas in a constructive manner
- Distributing content for research and to reinforce new concepts
- Asking targeted questions to a group or the class, focusing their attention on a specific aspect of the problem
Depending on your teaching style, it may take time to prepare yourself to successfully run a problem-based learning lesson.
4. Time-Consuming Assessment

If you choose to give marks, assessing a student’s performance throughout a problem-based learning exercise demands constant monitoring and note-taking. You must take factors into account such as:
- Completed tasks
- The quality of those tasks
- The group’s overall work and solution
- Communication among team members
- Anything you outlined on the activity’s rubric
Monitoring these criteria is required for each student, making it time-consuming to give and justify a mark for everyone.
5. Varying Degrees of Relevancy and Applicability
It can be difficult to identify a tangible problem that students can solve with content they’re studying and skills they’re mastering. This introduces two clear issues. First, if it is easy for students to divert from the challenge’s objectives, they may miss pertinent information. Second, you could veer off the problem’s focus and purpose as students run into unanticipated obstacles. Overcoming obstacles has benefits, but may compromise the planning you did. It can also make it hard to get back on track once the activity is complete. Because of the difficulty associated with keeping activities relevant and applicable, you may see problem-based learning as too taxing.
If the advantages outweigh the disadvantages -- or you just want to give problem-based learning a shot -- follow these steps:
1. Identify an Applicable Real-Life Problem

Find a tangible problem that’s relevant to your students, allowing them to easily contextualize it and hopefully apply it to future challenges. To identify an appropriate real-world problem, look at issues related to your:
- Students’ shared interests
You must also ensure that students understand the problem and the information around it. So, not all problems are appropriate for all grade levels.
2. Determine the Overarching Purpose of the Activity
Depending on the problem you choose, determine what you want to accomplish by running the challenge. For example, you may intend to help your students improve skills related to:
- Collaboration
- Problem-solving
- Curriculum-aligned topics
- Processing diverse content
A more precise example, you may prioritize collaboration skills by assigning specific tasks to pairs of students within each team. In doing so, students will continuously develop communication and collaboration abilities by working as a couple and part of a small group. By defining a clear purpose, you’ll also have an easier time following the next step.
3. Create and Distribute Helpful Material

Handouts and other content not only act as a set of resources, but help students stay focused on the activity and its purpose. For example, if you want them to improve a certain math skill , you should make material that highlights the mathematical aspects of the problem. You may decide to provide items such as:
- Data that helps quantify and add context to the problem
- Videos, presentations and other audio-visual material
- A list of preliminary questions to investigate
Providing a range of resources can be especially important for elementary students and struggling students in higher grades, who may not have self-direction skills to work without them.
4. Set Goals and Expectations for Your Students
Along with the aforementioned materials, give students a guide or rubric that details goals and expectations. It will allow you to further highlight the purpose of the problem-based learning exercise, as you can explain what you’re looking for in terms of collaboration, the final product and anything else. It should also help students stay on track by acting as a reference throughout the activity.
5. Participate

Although explicitly correcting students may be discouraged, you can still help them and ask questions to dig into their thought processes. When you see an opportunity, consider if it’s worthwhile to:
- Fill gaps in knowledge
- Provide hints, not answers
- Question a student’s conclusion or logic regarding a certain point, helping them think through tough spots
By participating in these ways, you can provide insight when students need it most, encouraging them to effectively analyze the problem.
6. Have Students Present Ideas and Findings
If you divided them into small groups, requiring students to present their thoughts and results in front the class adds a large-group learning component to the lesson. Encourage other students to ask questions, allowing the presenting group to elaborate and provide evidence for their thoughts. This wraps up the activity and gives your class a final chance to find solutions to the problem.
Wrapping Up
The effectiveness of problem-based learning may differ between classrooms and individual students, depending on how significant specific advantages and disadvantages are to you. Evaluative research consistently shows value in giving students a question and letting them take control of their learning. But the extent of this value can depend on the difficulties you face.It may be wise to try a problem-based learning activity, and go forward based on results.
Create or log into your teacher account on Prodigy -- an adaptive math game that adjusts content to accommodate player trouble spots and learning speeds. Aligned to US and Canadian curricula, it’s used by more than 350,000 teachers and 10 million students. It may be wise to try a problem-based learning activity, and go forward based on results.
Share this article
Table of Contents
Easily differentiate learning and engage your students with Prodigy Math.
Problem-Solving Method in Teaching
The problem-solving method is a highly effective teaching strategy that is designed to help students develop critical thinking skills and problem-solving abilities . It involves providing students with real-world problems and challenges that require them to apply their knowledge, skills, and creativity to find solutions. This method encourages active learning, promotes collaboration, and allows students to take ownership of their learning.
Table of Contents
Definition of problem-solving method.
Problem-solving is a process of identifying, analyzing, and resolving problems. The problem-solving method in teaching involves providing students with real-world problems that they must solve through collaboration and critical thinking. This method encourages students to apply their knowledge and creativity to develop solutions that are effective and practical.
Meaning of Problem-Solving Method
The meaning and Definition of problem-solving are given by different Scholars. These are-
Woodworth and Marquis(1948) : Problem-solving behavior occurs in novel or difficult situations in which a solution is not obtainable by the habitual methods of applying concepts and principles derived from past experience in very similar situations.
Skinner (1968): Problem-solving is a process of overcoming difficulties that appear to interfere with the attainment of a goal. It is the procedure of making adjustments in spite of interference
Benefits of Problem-Solving Method
The problem-solving method has several benefits for both students and teachers. These benefits include:
- Encourages active learning: The problem-solving method encourages students to actively participate in their own learning by engaging them in real-world problems that require critical thinking and collaboration
- Promotes collaboration: Problem-solving requires students to work together to find solutions. This promotes teamwork, communication, and cooperation.
- Builds critical thinking skills: The problem-solving method helps students develop critical thinking skills by providing them with opportunities to analyze and evaluate problems
- Increases motivation: When students are engaged in solving real-world problems, they are more motivated to learn and apply their knowledge.
- Enhances creativity: The problem-solving method encourages students to be creative in finding solutions to problems.
Steps in Problem-Solving Method
The problem-solving method involves several steps that teachers can use to guide their students. These steps include
- Identifying the problem: The first step in problem-solving is identifying the problem that needs to be solved. Teachers can present students with a real-world problem or challenge that requires critical thinking and collaboration.
- Analyzing the problem: Once the problem is identified, students should analyze it to determine its scope and underlying causes.
- Generating solutions: After analyzing the problem, students should generate possible solutions. This step requires creativity and critical thinking.
- Evaluating solutions: The next step is to evaluate each solution based on its effectiveness and practicality
- Selecting the best solution: The final step is to select the best solution and implement it.
Verification of the concluded solution or Hypothesis
The solution arrived at or the conclusion drawn must be further verified by utilizing it in solving various other likewise problems. In case, the derived solution helps in solving these problems, then and only then if one is free to agree with his finding regarding the solution. The verified solution may then become a useful product of his problem-solving behavior that can be utilized in solving further problems. The above steps can be utilized in solving various problems thereby fostering creative thinking ability in an individual.
The problem-solving method is an effective teaching strategy that promotes critical thinking, creativity, and collaboration. It provides students with real-world problems that require them to apply their knowledge and skills to find solutions. By using the problem-solving method, teachers can help their students develop the skills they need to succeed in school and in life.
- Jonassen, D. (2011). Learning to solve problems: A handbook for designing problem-solving learning environments. Routledge.
- Hmelo-Silver, C. E. (2004). Problem-based learning: What and how do students learn? Educational Psychology Review, 16(3), 235-266.
- Mergendoller, J. R., Maxwell, N. L., & Bellisimo, Y. (2006). The effectiveness of problem-based instruction: A comparative study of instructional methods and student characteristics. Interdisciplinary Journal of Problem-based Learning, 1(2), 49-69.
- Richey, R. C., Klein, J. D., & Tracey, M. W. (2011). The instructional design knowledge base: Theory, research, and practice. Routledge.
- Savery, J. R., & Duffy, T. M. (2001). Problem-based learning: An instructional model and its constructivist framework. CRLT Technical Report No. 16-01, University of Michigan. Wojcikowski, J. (2013). Solving real-world problems through problem-based learning. College Teaching, 61(4), 153-156

Center for Teaching Innovation
Resource library.
- Establishing Community Agreements and Classroom Norms
- Sample group work rubric
- Problem-Based Learning Clearinghouse of Activities, University of Delaware
Problem-Based Learning
Problem-based learning (PBL) is a student-centered approach in which students learn about a subject by working in groups to solve an open-ended problem. This problem is what drives the motivation and the learning.
Why Use Problem-Based Learning?
Nilson (2010) lists the following learning outcomes that are associated with PBL. A well-designed PBL project provides students with the opportunity to develop skills related to:
- Working in teams.
- Managing projects and holding leadership roles.
- Oral and written communication.
- Self-awareness and evaluation of group processes.
- Working independently.
- Critical thinking and analysis.
- Explaining concepts.
- Self-directed learning.
- Applying course content to real-world examples.
- Researching and information literacy.
- Problem solving across disciplines.
Considerations for Using Problem-Based Learning
Rather than teaching relevant material and subsequently having students apply the knowledge to solve problems, the problem is presented first. PBL assignments can be short, or they can be more involved and take a whole semester. PBL is often group-oriented, so it is beneficial to set aside classroom time to prepare students to work in groups and to allow them to engage in their PBL project.
Students generally must:
- Examine and define the problem.
- Explore what they already know about underlying issues related to it.
- Determine what they need to learn and where they can acquire the information and tools necessary to solve the problem.
- Evaluate possible ways to solve the problem.
- Solve the problem.
- Report on their findings.
Getting Started with Problem-Based Learning
- Articulate the learning outcomes of the project. What do you want students to know or be able to do as a result of participating in the assignment?
- Create the problem. Ideally, this will be a real-world situation that resembles something students may encounter in their future careers or lives. Cases are often the basis of PBL activities. Previously developed PBL activities can be found online through the University of Delaware’s PBL Clearinghouse of Activities .
- Establish ground rules at the beginning to prepare students to work effectively in groups.
- Introduce students to group processes and do some warm up exercises to allow them to practice assessing both their own work and that of their peers.
- Consider having students take on different roles or divide up the work up amongst themselves. Alternatively, the project might require students to assume various perspectives, such as those of government officials, local business owners, etc.
- Establish how you will evaluate and assess the assignment. Consider making the self and peer assessments a part of the assignment grade.
Nilson, L. B. (2010). Teaching at its best: A research-based resource for college instructors (2nd ed.). San Francisco, CA: Jossey-Bass.
- Effective Teaching Strategies
Problem-Based Learning: Benefits and Risks
- November 12, 2009
- Maryellen Weimer, PhD
Problem-based learning, the instructional approach in which carefully constructed, open-ended problems are used by groups of students to work through content to a solution, has gained a foothold in many segments of higher education.
Originally PBL, as it’s usually called, was used in medical school and in some business curricula for majors. But now it is being used in a wide range of disciplines and with students at various educational levels. The article (reference below) from which material is about to be cited “makes a critical assessment” of how PBL is being used in the field of geography.
Much of the content is relevant to that discipline specifically, but the article does contain a useful table that summarizes the benefits and risks of PBL for students, instructors, and institutions. Material on the table is gleaned from an extensive review of the literature (all referenced in the article). Here’s some of the information contained in the table.
Benefits of Problem-Based Learning
For Students
- It’s a student-centered approach.
- Typically students find it more enjoyable and satisfying.
- It encourages greater understanding.
- Students with PBL experience rate their abilities higher.
- PBL develops lifelong learning skills.
For Instructors
- Class attendance increases.
- The method affords more intrinsic reward.
- It encourages students to spend more time studying.
- It promotes interdisciplinarity.
For Institutions
- It makes student learning a priority.
- It may aid student retention.
- It may be taken as evidence that an institution values teaching.
Risks of Problem-Based Learning
- Prior learning experiences do not prepare students well for PBL.
- PBL requires more time and takes away study time from other subjects.
- It creates some anxiety because learning is messier.
- Sometimes group dynamics issues compromise PBL effectiveness.
- Less content knowledge may be learned.
- Creating suitable problem scenarios is difficult.
- It requires more prep time.
- Students have queries about the process.
- Group dynamics issues may require faculty intervention.
- It raises new questions about what to assess and how.
- It requires a change in educational philosophy for faculty who mostly lecture.
- Faculty will need staff development and support.
- It generally takes more instructors.
- It works best with flexible classroom space.
- It engenders resistance from faculty who question its efficacy.
Reference: Pawson, E., Fournier, E., Haight, M., Muniz, O., Trafford, J., and Vajoczki, S. 2006. Problem-based learning in geography: Towards a critical assessment of its purposes, benefits and risks. Journal of Geography in Higher Education 30 (1): 103–16.
Excerpted from The Teaching Professor , February 2007.
Stay Updated with Faculty Focus!
Get exclusive access to programs, reports, podcast episodes, articles, and more!
- Opens in a new tab
Welcome Back
Username or Email
Remember Me
Already a subscriber? log in here.
Center for Teaching
Teaching problem solving.
Print Version
Tips and Techniques
Expert vs. novice problem solvers, communicate.
- Have students identify specific problems, difficulties, or confusions . Don’t waste time working through problems that students already understand.
- If students are unable to articulate their concerns, determine where they are having trouble by asking them to identify the specific concepts or principles associated with the problem.
- In a one-on-one tutoring session, ask the student to work his/her problem out loud . This slows down the thinking process, making it more accurate and allowing you to access understanding.
- When working with larger groups you can ask students to provide a written “two-column solution.” Have students write up their solution to a problem by putting all their calculations in one column and all of their reasoning (in complete sentences) in the other column. This helps them to think critically about their own problem solving and helps you to more easily identify where they may be having problems. Two-Column Solution (Math) Two-Column Solution (Physics)
Encourage Independence
- Model the problem solving process rather than just giving students the answer. As you work through the problem, consider how a novice might struggle with the concepts and make your thinking clear
- Have students work through problems on their own. Ask directing questions or give helpful suggestions, but provide only minimal assistance and only when needed to overcome obstacles.
- Don’t fear group work ! Students can frequently help each other, and talking about a problem helps them think more critically about the steps needed to solve the problem. Additionally, group work helps students realize that problems often have multiple solution strategies, some that might be more effective than others
Be sensitive
- Frequently, when working problems, students are unsure of themselves. This lack of confidence may hamper their learning. It is important to recognize this when students come to us for help, and to give each student some feeling of mastery. Do this by providing positive reinforcement to let students know when they have mastered a new concept or skill.
Encourage Thoroughness and Patience
- Try to communicate that the process is more important than the answer so that the student learns that it is OK to not have an instant solution. This is learned through your acceptance of his/her pace of doing things, through your refusal to let anxiety pressure you into giving the right answer, and through your example of problem solving through a step-by step process.
Experts (teachers) in a particular field are often so fluent in solving problems from that field that they can find it difficult to articulate the problem solving principles and strategies they use to novices (students) in their field because these principles and strategies are second nature to the expert. To teach students problem solving skills, a teacher should be aware of principles and strategies of good problem solving in his or her discipline .
The mathematician George Polya captured the problem solving principles and strategies he used in his discipline in the book How to Solve It: A New Aspect of Mathematical Method (Princeton University Press, 1957). The book includes a summary of Polya’s problem solving heuristic as well as advice on the teaching of problem solving.

Teaching Guides
- Online Course Development Resources
- Principles & Frameworks
- Pedagogies & Strategies
- Reflecting & Assessing
- Challenges & Opportunities
- Populations & Contexts
Quick Links
- Services for Departments and Schools
- Examples of Online Instructional Modules
Teaching Problem-Solving Skills
Many instructors design opportunities for students to solve “problems”. But are their students solving true problems or merely participating in practice exercises? The former stresses critical thinking and decision making skills whereas the latter requires only the application of previously learned procedures.
Problem solving is often broadly defined as "the ability to understand the environment, identify complex problems, review related information to develop, evaluate strategies and implement solutions to build the desired outcome" (Fissore, C. et al, 2021). True problem solving is the process of applying a method – not known in advance – to a problem that is subject to a specific set of conditions and that the problem solver has not seen before, in order to obtain a satisfactory solution.
Below you will find some basic principles for teaching problem solving and one model to implement in your classroom teaching.
Principles for teaching problem solving
- Model a useful problem-solving method . Problem solving can be difficult and sometimes tedious. Show students how to be patient and persistent, and how to follow a structured method, such as Woods’ model described below. Articulate your method as you use it so students see the connections.
- Teach within a specific context . Teach problem-solving skills in the context in which they will be used by students (e.g., mole fraction calculations in a chemistry course). Use real-life problems in explanations, examples, and exams. Do not teach problem solving as an independent, abstract skill.
- Help students understand the problem . In order to solve problems, students need to define the end goal. This step is crucial to successful learning of problem-solving skills. If you succeed at helping students answer the questions “what?” and “why?”, finding the answer to “how?” will be easier.
- Take enough time . When planning a lecture/tutorial, budget enough time for: understanding the problem and defining the goal (both individually and as a class); dealing with questions from you and your students; making, finding, and fixing mistakes; and solving entire problems in a single session.
- Ask questions and make suggestions . Ask students to predict “what would happen if …” or explain why something happened. This will help them to develop analytical and deductive thinking skills. Also, ask questions and make suggestions about strategies to encourage students to reflect on the problem-solving strategies that they use.
- Link errors to misconceptions . Use errors as evidence of misconceptions, not carelessness or random guessing. Make an effort to isolate the misconception and correct it, then teach students to do this by themselves. We can all learn from mistakes.
Woods’ problem-solving model
Define the problem.
- The system . Have students identify the system under study (e.g., a metal bridge subject to certain forces) by interpreting the information provided in the problem statement. Drawing a diagram is a great way to do this.
- Known(s) and concepts . List what is known about the problem, and identify the knowledge needed to understand (and eventually) solve it.
- Unknown(s) . Once you have a list of knowns, identifying the unknown(s) becomes simpler. One unknown is generally the answer to the problem, but there may be other unknowns. Be sure that students understand what they are expected to find.
- Units and symbols . One key aspect in problem solving is teaching students how to select, interpret, and use units and symbols. Emphasize the use of units whenever applicable. Develop a habit of using appropriate units and symbols yourself at all times.
- Constraints . All problems have some stated or implied constraints. Teach students to look for the words "only", "must", "neglect", or "assume" to help identify the constraints.
- Criteria for success . Help students consider, from the beginning, what a logical type of answer would be. What characteristics will it possess? For example, a quantitative problem will require an answer in some form of numerical units (e.g., $/kg product, square cm, etc.) while an optimization problem requires an answer in the form of either a numerical maximum or minimum.
Think about it
- “Let it simmer”. Use this stage to ponder the problem. Ideally, students will develop a mental image of the problem at hand during this stage.
- Identify specific pieces of knowledge . Students need to determine by themselves the required background knowledge from illustrations, examples and problems covered in the course.
- Collect information . Encourage students to collect pertinent information such as conversion factors, constants, and tables needed to solve the problem.
Plan a solution
- Consider possible strategies . Often, the type of solution will be determined by the type of problem. Some common problem-solving strategies are: compute; simplify; use an equation; make a model, diagram, table, or chart; or work backwards.
- Choose the best strategy . Help students to choose the best strategy by reminding them again what they are required to find or calculate.
Carry out the plan
- Be patient . Most problems are not solved quickly or on the first attempt. In other cases, executing the solution may be the easiest step.
- Be persistent . If a plan does not work immediately, do not let students get discouraged. Encourage them to try a different strategy and keep trying.
Encourage students to reflect. Once a solution has been reached, students should ask themselves the following questions:
- Does the answer make sense?
- Does it fit with the criteria established in step 1?
- Did I answer the question(s)?
- What did I learn by doing this?
- Could I have done the problem another way?
If you would like support applying these tips to your own teaching, CTE staff members are here to help. View the CTE Support page to find the most relevant staff member to contact.
- Fissore, C., Marchisio, M., Roman, F., & Sacchet, M. (2021). Development of problem solving skills with Maple in higher education. In: Corless, R.M., Gerhard, J., Kotsireas, I.S. (eds) Maple in Mathematics Education and Research. MC 2020. Communications in Computer and Information Science, vol 1414. Springer, Cham. https://doi.org/10.1007/978-3-030-81698-8_15
- Foshay, R., & Kirkley, J. (1998). Principles for Teaching Problem Solving. TRO Learning Inc., Edina MN. (PDF) Principles for Teaching Problem Solving (researchgate.net)
- Hayes, J.R. (1989). The Complete Problem Solver. 2nd Edition. Hillsdale, NJ: Lawrence Erlbaum Associates.
- Woods, D.R., Wright, J.D., Hoffman, T.W., Swartman, R.K., Doig, I.D. (1975). Teaching Problem solving Skills.
- Engineering Education. Vol 1, No. 1. p. 238. Washington, DC: The American Society for Engineering Education.
Catalog search
Teaching tip categories.
- Assessment and feedback
- Blended Learning and Educational Technologies
- Career Development
- Course Design
- Course Implementation
- Inclusive Teaching and Learning
- Learning activities
- Support for Student Learning
- Support for TAs
- Learning activities ,
Why Every Educator Needs to Teach Problem-Solving Skills
Strong problem-solving skills will help students be more resilient and will increase their academic and career success .
Want to learn more about how to measure and teach students’ higher-order skills, including problem solving, critical thinking, and written communication?
Problem-solving skills are essential in school, careers, and life.
Problem-solving skills are important for every student to master. They help individuals navigate everyday life and find solutions to complex issues and challenges. These skills are especially valuable in the workplace, where employees are often required to solve problems and make decisions quickly and effectively.
Problem-solving skills are also needed for students’ personal growth and development because they help individuals overcome obstacles and achieve their goals. By developing strong problem-solving skills, students can improve their overall quality of life and become more successful in their personal and professional endeavors.
Problem-Solving Skills Help Students…
develop resilience.
Problem-solving skills are an integral part of resilience and the ability to persevere through challenges and adversity. To effectively work through and solve a problem, students must be able to think critically and creatively. Critical and creative thinking help students approach a problem objectively, analyze its components, and determine different ways to go about finding a solution.
This process in turn helps students build self-efficacy . When students are able to analyze and solve a problem, this increases their confidence, and they begin to realize the power they have to advocate for themselves and make meaningful change.
When students gain confidence in their ability to work through problems and attain their goals, they also begin to build a growth mindset . According to leading resilience researcher, Carol Dweck, “in a growth mindset, people believe that their most basic abilities can be developed through dedication and hard work—brains and talent are just the starting point. This view creates a love of learning and a resilience that is essential for great accomplishment.”
Set and Achieve Goals
Students who possess strong problem-solving skills are better equipped to set and achieve their goals. By learning how to identify problems, think critically, and develop solutions, students can become more self-sufficient and confident in their ability to achieve their goals. Additionally, problem-solving skills are used in virtually all fields, disciplines, and career paths, which makes them important for everyone. Building strong problem-solving skills will help students enhance their academic and career performance and become more competitive as they begin to seek full-time employment after graduation or pursue additional education and training.
Resolve Conflicts
In addition to increased social and emotional skills like self-efficacy and goal-setting, problem-solving skills teach students how to cooperate with others and work through disagreements and conflicts. Problem-solving promotes “thinking outside the box” and approaching a conflict by searching for different solutions. This is a very different (and more effective!) method than a more stagnant approach that focuses on placing blame or getting stuck on elements of a situation that can’t be changed.
While it’s natural to get frustrated or feel stuck when working through a conflict, students with strong problem-solving skills will be able to work through these obstacles, think more rationally, and address the situation with a more solution-oriented approach. These skills will be valuable for students in school, their careers, and throughout their lives.
Achieve Success
We are all faced with problems every day. Problems arise in our personal lives, in school and in our jobs, and in our interactions with others. Employers especially are looking for candidates with strong problem-solving skills. In today’s job market, most jobs require the ability to analyze and effectively resolve complex issues. Students with strong problem-solving skills will stand out from other applicants and will have a more desirable skill set.
In a recent opinion piece published by The Hechinger Report , Virgel Hammonds, Chief Learning Officer at KnowledgeWorks, stated “Our world presents increasingly complex challenges. Education must adapt so that it nurtures problem solvers and critical thinkers.” Yet, the “traditional K–12 education system leaves little room for students to engage in real-world problem-solving scenarios.” This is the reason that a growing number of K–12 school districts and higher education institutions are transforming their instructional approach to personalized and competency-based learning, which encourage students to make decisions, problem solve and think critically as they take ownership of and direct their educational journey.
Problem-Solving Skills Can Be Measured and Taught
Research shows that problem-solving skills can be measured and taught. One effective method is through performance-based assessments which require students to demonstrate or apply their knowledge and higher-order skills to create a response or product or do a task.
What Are Performance-Based Assessments?

With the No Child Left Behind Act (2002), the use of standardized testing became the primary way to measure student learning in the U.S. The legislative requirements of this act shifted the emphasis to standardized testing, and this led to a decline in nontraditional testing methods .
But many educators, policy makers, and parents have concerns with standardized tests. Some of the top issues include that they don’t provide feedback on how students can perform better, they don’t value creativity, they are not representative of diverse populations, and they can be disadvantageous to lower-income students.
While standardized tests are still the norm, U.S. Secretary of Education Miguel Cardona is encouraging states and districts to move away from traditional multiple choice and short response tests and instead use performance-based assessment, competency-based assessments, and other more authentic methods of measuring students abilities and skills rather than rote learning.
Performance-based assessments measure whether students can apply the skills and knowledge learned from a unit of study. Typically, a performance task challenges students to use their higher-order skills to complete a project or process. Tasks can range from an essay to a complex proposal or design.
Preview a Performance-Based Assessment
Want a closer look at how performance-based assessments work? Preview CAE’s K–12 and Higher Education assessments and see how CAE’s tools help students develop critical thinking, problem-solving, and written communication skills.
Performance-Based Assessments Help Students Build and Practice Problem-Solving Skills
In addition to effectively measuring students’ higher-order skills, including their problem-solving skills, performance-based assessments can help students practice and build these skills. Through the assessment process, students are given opportunities to practically apply their knowledge in real-world situations. By demonstrating their understanding of a topic, students are required to put what they’ve learned into practice through activities such as presentations, experiments, and simulations.
This type of problem-solving assessment tool requires students to analyze information and choose how to approach the presented problems. This process enhances their critical thinking skills and creativity, as well as their problem-solving skills. Unlike traditional assessments based on memorization or reciting facts, performance-based assessments focus on the students’ decisions and solutions, and through these tasks students learn to bridge the gap between theory and practice.
Performance-based assessments like CAE’s College and Career Readiness Assessment (CRA+) and Collegiate Learning Assessment (CLA+) provide students with in-depth reports that show them which higher-order skills they are strongest in and which they should continue to develop. This feedback helps students and their teachers plan instruction and supports to deepen their learning and improve their mastery of critical skills.

Explore CAE’s Problem-Solving Assessments
CAE offers performance-based assessments that measure student proficiency in higher-order skills including problem solving, critical thinking, and written communication.
- College and Career Readiness Assessment (CCRA+) for secondary education and
- Collegiate Learning Assessment (CLA+) for higher education.
Our solution also includes instructional materials, practice models, and professional development.
We can help you create a program to build students’ problem-solving skills that includes:
- Measuring students’ problem-solving skills through a performance-based assessment
- Using the problem-solving assessment data to inform instruction and tailor interventions
- Teaching students problem-solving skills and providing practice opportunities in real-life scenarios
- Supporting educators with quality professional development
Get started with our problem-solving assessment tools to measure and build students’ problem-solving skills today! These skills will be invaluable to students now and in the future.

Ready to Get Started?
Learn more about cae’s suite of products and let’s get started measuring and teaching students important higher-order skills like problem solving..

Simple Guide to Problem-Solving Method of Teaching
You must be interested to know – What is the problem-solving method of teaching and how it works. We’ve explained its core principles, six-step process, and benefits with real-world examples.
Understand the Problem-Solving Method of Teaching
The basis of this modern teaching approach is to provide students with opportunities to face real-time challenges. It aims to help them understand how the concept behind a solution works in reality.
What is the Problem-Solving Method of Teaching?
The problem-solving method of teaching is a student-centered approach to learning that focuses on developing students’ problem-solving skills. In this method, students have to face real-world problems to solve.
They are encouraged to use their knowledge and skills to provide solutions. The teacher acts as a facilitator, providing guidance and support as needed, but ultimately the students are responsible for finding their solutions.
Must Read: How to Tell Me About Yourself in an Interview
5 Most Important Benefits of Problem-Solving Method of Teaching
The new way of teaching primarily helps students develop critical thinking skills and real-world application abilities. It also promotes independence and self-confidence in problem-solving.
The problem-solving method of teaching has several benefits. It helps students to:
#1 Enhances critical thinking
By presenting students with real-world problems to solve, the problem-solving method of teaching forces them:
– To think critically about the situation, and – To come up with their solutions.
This process helps students develop critical thinking skills essential for success in school and life.

#2 Fosters creativity
The problem-solving method of teaching encourages students to be creative in their problem-solving approach. There is often no one right answer to a problem, so students are free to come up with their unique solutions. This process helps students think creatively, an important skill in all areas of life.
#3 Encourages real-world application
The problem-solving method of teaching helps students learn how to apply their knowledge to real-world situations. By solving real-world problems, students can see:
– How their knowledge is relevant to their lives, – And, the world around them.
This helps students to become more motivated and engaged learners.
#4 Builds student confidence
When students can successfully solve problems, they gain confidence in their abilities. This confidence is essential for success in all areas of life, both academic and personal.
#5 Promotes collaborative learning
The problem-solving method of teaching often involves students working together to solve problems. This collaborative learning process helps students to develop their teamwork skills and to learn from each other.
Know 6 Steps in the Problem-Solving Method of Teaching
Also Read: Do You Know the Difference Between ChatGPT and GPT-4?
The problem-solving method of teaching typically involves the following steps:
Step 1: Identifying the problem
The first step is problem identification which students will be working on. This requires students to do the following:
– By presenting students with a real-world problem, or – By asking them to come up with their problems.
Step 2: Understanding the problem
Once students have identified the problem, they need to understand it fully. This may involve:
– Breaking the problem down into smaller parts, or – Gathering more information about the problem.
Step 3: Generating solutions
Once students understand the problem, they need to generate possible solutions. They have to do either of the following:
– By brainstorming, or – By exercising problem-solving techniques such as root cause analysis or the decision matrix.
Step 4: Evaluating solutions
Students need to evaluate the pros and cons of each solution before choosing one to implement.
Step 5: Implementing the solution
Once students have chosen a solution, they need to implement it. This may involve taking action or developing a plan.
Step 6: Evaluating the results
Once students have implemented the solution, they must evaluate the results to see if it was successful.
If the solution fails the expectations, students should re-run step 3 and generate new solutions.
Find Out Examples of the Problem-Solving Method of Teaching
Here are a few examples of how the problem-solving method of teaching applies to different subjects:
- Math: Students face real-world problems such as budgeting for a family or designing a new product. Students would then need to use their math skills to solve the problem.
- Science: Students perform a science experiment or research on a scientific topic to invent a solution to the problem. Students should then use their science knowledge and skills to solve the problem.
- Social studies: Students analyze a historical event or current social issue and devise a solution. After that, students should exercise their social studies knowledge and skills to solve the problem.
How to Use Problem-Solving Methods of Teaching
Here are a few tips for using the problem-solving method of teaching effectively:
- Choose problems that are relevant to students’ lives and interests.
- Select those problems that are challenging but achievable.
- Provide students with ample resources such as books, websites, or experts to solve the problem.
- Motivate them to work collaboratively and to share their ideas.
- Be patient and supportive. Problem-solving can be a challenging process, but it is also a rewarding one.
Also Try: 1-10 Random Number Generator
How to Choose: Let’s Draw a Comparison
The following table compares the different problem-solving methods:
| Method | Description | Pros | Cons |
|---|---|---|---|
| The teacher presents information to students who then complete exercises or assignments to practice the information. | – Simple and easy-to-follow | – Can be passive and boring for students | |
| Students are presented with real-world problems to solve. They are encouraged to use their knowledge and skills to deliver solutions. | – Promotes active learning | – Can be challenging for students | |
| Students are asked to investigate questions or problems. They are encouraged to gather evidence and come up with their conclusions. | – Encourages critical thinking | – Can be time-consuming |
Which Method is the Most Suitable?
The most suitable way of teaching will depend on many factors such as the following:
– Subject matter, – Student’s age and ability level, and – Teacher’s preferences.
However, the problem-solving method of teaching is a valuable approach. It can be used in any subject area and with students of all ages.
Here are some additional tips for using the problem-solving method of teaching effectively:
- Differentiate instruction. Not all students learn at the same pace or in the same way. Teachers can differentiate instruction to meet the needs of all learners by providing different levels of support and scaffolding.
- Use formative assessment. Formative assessment helps track students’ progress and identify areas where they need additional support. Teachers can then use this information to provide students with targeted instruction.
- Create a positive learning environment. Students need to feel safe and supported to learn effectively. Teachers can create a positive learning environment by providing students with opportunities for collaboration. They can celebrate their successes and create a classroom culture where mistakes are seen as learning opportunities.
Interested in New Tech: 7 IoT Trends to Watch in 2023
Some Unique Examples to Refer to Before We Conclude
Here are a few unique examples of how you incorporate the problem-solving method of teaching with different subjects:
- English: Students analyze a grammar problem, such as a poem or a short story, and share their interpretation.
- Art: Students can get a task to design a new product or to create a piece of art that addresses a social issue.
- Music: Students write a song about a current event or create a new piece of music reflecting their cultural heritage.
Before You Leave
The problem-solving method of teaching is a powerful tool that can help students develop the skills they need to succeed in school and life. By creating a learning environment where students are encouraged to think critically and solve problems, teachers can help students to become lifelong learners.
Lastly, our site needs your support to remain free. Share this post on social media ( Linkedin / Twitter ) if you gained some knowledge from this tutorial.
Enjoy learning, TechBeamers.
You Might Also Like
Difference between spring and spring boot, generate 1-10 random number online, how to speed up your website to load faster, directory in computer aside from git bash github, best ides for golang in 2024, leave a reply.
Your email address will not be published. Required fields are marked *
Popular Tutorials
ORIGINAL RESEARCH article
The problem-solving method: efficacy for learning and motivation in the field of physical education.

- 1 High Institute of Sport and Physical Education of Sfax, University of Sfax, Sfax, Tunisia
- 2 Research Unit of the National Sports Observatory (ONS), Tunis, Tunisia
- 3 Research Laboratory: Education, Motricity, Sport and Health, EM2S, LR19JS01, University of Sfax, Sfax, Tunisia
- 4 Department of Neuroscience, Rehabilitation, Ophthalmology, Genetics, Maternal and Child Health (DINOGMI), University of Genoa, Genoa, Italy
- 5 Centre for Intelligent Healthcare, Coventry University, Coventry, United Kingdom
- 6 Laboratory for Industrial and Applied Mathematics, Department of Mathematics and Statistics, York University, Toronto, ON, Canada
- 7 High Institute of Sport and Physical Education of Ksar Saîd, University Manouba, UMA, Manouba, Tunisia
Background: In pursuit of quality teaching and learning, teachers seek the best method to provide their students with a positive educational atmosphere and the most appropriate learning conditions.
Objectives: The purpose of this study is to compare the effects of the problem-solving method vs. the traditional method on motivation and learning during physical education courses.
Methods: Fifty-three students ( M age 15 ± 0.1 years), in their 1st year of the Tunisian secondary education system, voluntarily participated in this study, and randomly assigned to a control or experimental group. Participants in the control group were taught using the traditional methods, whereas participants in the experimental group were taught using the problem-solving method. Both groups took part in a 10-hour experiment over 5 weeks. To measure students' situational motivation, a questionnaire was used to evaluate intrinsic motivation, identified regulation, external regulation, and amotivation during the first (T0) and the last sessions (T2). Additionally, the degree of students' learning was determined via video analyses, recorded at T0, the fifth (T1), and T2.
Results: Motivational dimensions, including identified regulation and intrinsic motivation, were significantly greater (all p < 0.001) in the experimental vs. the control group. The students' motor engagement in learning situations, during which the learner, despite a degree of difficulty performs the motor activity with sufficient success, increased only in the experimental group ( p < 0.001). The waiting time in the experimental group decreased significantly at T1 and T2 vs. T0 (all p < 0.001), with lower values recorded in the experimental vs. the control group at the three-time points (all p < 0.001).
Conclusions: The problem-solving method is an efficient strategy for motor skills and performance enhancement, as well as motivation development during physical education courses.
1. Introduction
The education of children is a sensitive and poignant subject, where the wellbeing of the child in the school environment is a key issue ( Ergül and Kargin, 2014 ). For this, numerous research has sought to find solutions to the problems of the traditional method, which focuses on the teacher as an instructor, giver of knowledge, arbiter of truth, and ultimate evaluator of learning ( Ergül and Kargin, 2014 ; Cunningham and Sood, 2018 ). From this perspective, a teachers' job is to present students with a designated body of knowledge in a predetermined order ( Arvind and Kusum, 2017 ). For them, learners are seen as people with “knowledge gaps” that need to be filled with information. In this method, teaching is conceived as the act of transmitting knowledge from point A (responsible for the teacher) to point B (responsible for the students; Arvind and Kusum, 2017 ). According to Novak (2010) , in the traditional method, the teacher is the one who provokes the learning.
The traditional method focuses on lecture-based teaching as the center of instruction, emphasizing delivery of program and concept ( Johnson, 2010 ; Ilkiw et al., 2017 ; Dickinson et al., 2018 ). The student listens and takes notes, passively accepts and receives from the teacher undifferentiated and identical knowledge ( Bi et al., 2019 ). Course content and delivery are considered most important, and learners acquire knowledge through exercise and practice ( Johnson et al., 1998 ). In the traditional method, academic achievement is seen as the ability of students to demonstrate, replicate, or convey this designated body of knowledge to the teacher. It is based on a transmissive model, the teacher contenting themselves with exchanging and transmitting information to the learner. Here, only the “knowledge” and “teacher” poles of the pedagogical triangle are solicited. The teacher teaches the students, who play the role of the spectator. They receive information without participating in its creation ( Perrenoud, 2003 ). For this, researchers invented a new student-centered method with effects on improving students' graphic interpretation skills and conceptual understanding of kinematic motion represent an area of contemporary interest ( Tebabal and Kahssay, 2011 ). Indeed, in order to facilitate the process of knowledge transfer, teachers should use appropriate methods targeted to specific objectives of the school curricula.
For instance, it has been emphasized that the effectiveness of any educational process as a whole relies on the crucial role of using a well-designed pedagogical (teaching and/or learning) strategy ( Kolesnikova, 2016 ).
Alternate to a traditional method of teaching, Ergül and Kargin (2014 ), proposed the problem-solving method, which represents one of the most common student-centered learning strategies. Indeed, this method allows students to participate in the learning environment, giving them the responsibility for their own acquisition of knowledge, as well as the opportunity for the understanding and structuring of diverse information.
For Cunningham and Sood (2018) , the problem-solving method may be considered a fundamental tool for the acquisition of new knowledge, notably learning transfer. Moreover, the problem-solving method is purportedly efficient for the development of manual skills and experiential learning ( Ergül and Kargin, 2014 ), as well as the optimization of thinking ability. Additionally, the problem-solving method allows learners to participate in the learning environment, while giving them responsibility for their learning and making them understand and structure the information ( Pohan et al., 2020 ). In this context, Ali (2019) reported that, when faced with an obstacle, the student will have to invoke his/her knowledge and use his/her abilities to “break the deadlock.” He/she will therefore make the most of his/her potential, but also share and exchange with his/her colleagues ( Ali, 2019 ). Throughout the process, the student will learn new concepts and skills. The role of the teacher is paramount at the beginning of the activity, since activities will be created based on problematic situations according to the subject and the program. However, on the day of the activity, it does not have the main role, and the teacher will guide learners in difficulty and will allow them to manage themselves most of the time ( Ali, 2019 ).
The problem-solving method encourages group discussion and teamwork ( Fidan and Tuncel, 2019 ). Additionally, in this pedagogical approach, the role of the teacher is a facilitator of learning, and they take on a much more interactive and less rebarbative role ( Garrett, 2008 ).
For the teaching method to be effective, teaching should consist of an ongoing process of making desirable changes among learners using appropriate methods ( Ayeni, 2011 ; Norboev, 2021 ). To bring about positive changes in students, the methods used by teachers should be the best for the subject to be taught ( Adunola et al., 2012 ). Further, suggests that teaching methods work effectively, especially if they meet the needs of learners since each learner interprets and answers questions in a unique way. Improving problem-solving skills is a primary educational goal, as is the ability to use reasoning. To acquire this skill, students must solve problems to learn mathematics and problem-solving ( Hu, 2010 ); this encourages the students to actively participate and contribute to the activities suggested by the teacher. Without sufficient motivation, learning goals can no longer be optimally achieved, although learners may have exceptional abilities. The method of teaching employed by the teachers is decisive to achieve motivational consequences in physical education students ( Leo et al., 2022 ). Pérez-Jorge et al. (2021 ) posited that given we now live in a technological society in which children are used to receiving a large amount of stimuli, gaining and maintaining their attention and keeping them motivated at school becomes a challenge for teachers.
Fenouillet (2012) stated that academic motivation is linked to resources and methods that improve attention for school learning. Furthermore, Rolland (2009) and Bessa et al. (2021) reported a link between a learner's motivational dynamics and classroom activities. The models of learning situations, where the student is the main actor, directly refers to active teaching methods, and that there is a strong link between motivation and active teaching ( Rossa et al., 2021 ). In the same context, previous reports assert that the motivation of students in physical education is an important factor since the intra-individual motivation toward this discipline is recognized as a major determinant of physical activity for students ( Standage et al., 2012 ; Luo, 2019 ; Leo et al., 2022 ). Further, extensive research on the effectiveness of teaching methods shows that the quality of teaching often influences the performance of learners ( Norboev, 2021 ). Ayeni (2011) reported that education is a process that allows students to make changes desirable to achieve specific results. Thus, the consistency of teaching methods with student needs and learning influences student achievement. This has led several researchers to explore the impact of different teaching strategies, ranging from traditional methods to active learning techniques that can be used such as the problem-solving method ( Skinner, 1985 ; Darling-Hammond et al., 2020 ).
In the context of innovation, Blázquez (2016 ) emphasizes the importance of adopting active methods and implementing them as the main element promoting the development of skills, motivation and active participation. Pedagogical models are part of the active methods which, together with model-based practice, replace traditional teaching ( Hastie and Casey, 2014 ; Casey et al., 2021 ). Thus, many studies have identified pedagogical models as the most effective way to place students at the center of the teaching-learning process ( Metzler, 2017 ), making it possible to assess the impact of physical education on learning students ( Casey, 2014 ; Rivera-Pérez et al., 2020 ; Manninen and Campbell, 2021 ). Since each model is designed to focus on a specific program objective, each model has limitations when implemented in isolation ( Bunker and Thorpe, 1982 ; Rivera-Pérez et al., 2020 ). Therefore, focusing on developing students' social and emotional skills and capacities could help them avoid failure in physical education ( Ang and Penney, 2013 ). Thus, the current emergence of new pedagogical models goes with their hybridization with different methods, which is a wave of combinations proposed today as an innovative pedagogical strategy. The incorporation of this type of method in the current education system is becoming increasingly important because it gives students a greater role, participation, autonomy and self-regulation, and above all it improves their motivation ( Puigarnau et al., 2016 ). The teaching model of personal and social responsibility, for example, is closely related to the sports education model because both share certain approaches to responsibility ( Siedentop et al., 2011 ). One of the first studies to use these two models together was Rugby ( Gordon and Doyle, 2015 ), which found significant improvements in student behavior. Also, the recent study by Menendez and Fernandez-Rio (2017) on educational kickboxing.
Previous studies have indicated that hybridization can increase play, problem solving performance and motor skills ( Menendez and Fernandez-Rio, 2017 ; Ward et al., 2021 ) and generate positive psychosocial consequences, such as pleasure, intention to be physically active and responsibility ( Dyson and Grineski, 2001 ; Menendez and Fernandez-Rio, 2017 ).
But despite all these research results, the picture remains unclear, and it remains unknown which method is more effective in improving students' learning and motivation. Given the lack of published evidence on this topic, the aim of this study was to compare the effects of problem-solving vs. the traditional method on students' motivation and learning.
We hypothesized would that the problem-solving method would be more effective in improving students' motivation and learning better than the traditional method.
2. Materials and method
2.1. participants.
Fifty-three students, aged 15–16 ( M age 15 ± 0.1 years), in their 1st year of the Tunisian secondary education system, voluntarily participated in this study. All participants were randomly chosen. Repeating students, those who practice handball activity in civil/competitive/amateur clubs or in the high school sports association, and students who were absent, even for one session, were excluded. The first class consisted of 30 students (16 boys and 14 girls), who represented the experimental group and followed basic courses on a learning method by solving problems. The second class consisted of 23 students (10 boys and 13 girls), who represented the control group and followed the traditional teaching method. The total duration was spread over 5 weeks, or two sessions per week and each session lasted 50 min.
University research ethics board approval (CPPSUD: 0295/2021) was obtained before recruiting participants who were subsequently informed of the nature, objective, methodology, and constraints. Teacher, school director, parental/guardian, and child informed consent was obtained prior to participation in the study.
2.2. Procedure
Before the start of the experiment, the participants were familiarized with the equipment and the experimental protocol in order to ensure a good learning climate. For this and to mitigate the impact of the observer and the cameras on the students, the two researchers were involved prior to the data collection in a week of familiarization by making test recordings with the classes concerned.
An approach of a teaching cycle consisting of 10 sessions spread over 5 weeks, amounting to two sessions per week. Physical education classes were held in the morning from 8 a.m. to 9 a.m., with a single goal for each session that lasted 50 min. The cyclic programs were produced by the teacher responsible for carrying out the experiment with 18 years of service. To do this, the students had the same lessons with the same objectives, only pedagogy that differs: the experimental group worked using problem-solving pedagogy, while the control group was confronted with traditional pedagogy. The sessions took place in a handball field 40 m long and 20 m wide. Examples of training sessions using the problem-solving pedagogy and the traditional pedagogy are presented in Table 1 . In addition, a motivation questionnaire, the Situational Motivation Scale (SIMS; Guay et al., 2000 ), was administered to learners at the end of the session (i.e., in the beginning, and end of the cycle). Each student answered the questions alone and according to their own ideas. This questionnaire was taken in a classroom to prevent students from acting abnormally during the study. It lasted for a maximum of 10 min.

Table 1 . Example of activities for the different sessions.
Two diametrically opposed cameras were installed so to film all the movements and behaviors of each student and teacher during the three sessions [(i) test at the start of the cycle (T0), (ii) in the middle of the cycle (T1), and (iii) test at the end of the cycle (T2)]. These sessions had the same content and each consisted of four phases: the getting started, the warm-up, the work up (which consisted of three situations: first, the work was goes up the ball to two to score in the goal following a shot. Second, the same principle as the previous situation but in the presence of a defender. Finally, third, a match 7 ≠ 7), and the cooling down These recordings were analyzed using a Learning Time Analysis System grid (LTAS; Brunelle et al., 1988 ). This made it possible to measure individual learning by coding observable variables of the behavior of learners in a learning situation.
2.3. Data collection and analysis
2.3.1. the motivation questionnaire.
In this study, in order to measure the situational motivation of students, the situational motivation scale (SIMS; Guay et al., 2000 ), which used. This questionnaire assesses intrinsic motivation, identified regulation, external regulation and amotivation. SIMS has demonstrated good reliability and factor validity in the context of physical education in adolescents ( Lonsdale et al., 2011 ). The participants received exact instructions from the researchers in accordance with written instructions on how to conduct the data collection. Participants completed the SIMS anonymously at the start of a physical education class. All students had the opportunity to write down their answers without being observed and to ask questions if anything was unclear. To minimize the tendency to give socially desirable answers, they were asked to answer as honestly as possible, with the confidence that the teacher would not be able to read their answers and that their grades would not be affected by how they responded. The SIMS questionnaire was filled at T0 and T2. This scale is made up of 16 items divided into four dimensions: intrinsic motivation, identified regulation, external regulation and amotivation. Each item is rated on a 7-point Likert scale ranging from 1 (which is the weakest factor) “not at all” to 7 (which is the strongest factor) “exactly matches.”
In order to assess the internal consistency of the scales, a Cronbach alpha test was conducted ( Cronbach, 1951 ). The internal consistency of the scales was acceptable with reliability coefficients ranging from 0.719 to 0.87. The coefficient of reliability was 0.8.
In the present study, Cronbach's alphas were: intrinsic motivation = 0.790; regulation identified = 0.870; external regulation = 0.749; and amotivation = 0.719.
2.3.2. Camcorders
The audio-visual data collection was conducted using two Sony camcorders (Model; Handcam 4K) with a wireless microphone with a DJ transmitter-receiver (VHF 10HL F4 Micro HF) with a range of 80 m ( Maddeh et al., 2020 ). The collection took place over a period of 5 weeks, with three captures for each class (three sessions of 50 min for each at T0, T1, and T2). Two researchers were trained in the procedures and video capture techniques. The cameras were positioned diagonally, in order to film all the behavior of the students and teacher on the set.
2.3.3. The Learning Time Analysis System (LTAS)
To measure the degree of student learning, the analysis of videos recorded using the LTAS grid by Brunelle et al. (1988) was used, at T0, T1, and T2. This observation system with predetermined categories uses the technique of observation by small intervals (i.e., 6 s) and allows to measure individual learning by coding observable variables of their behaviors when they have been in a learning situation. This grid also permits the specification of the quantity and quality with which the participants engaged in the requested work and was graded, broadly, on two characteristics: the type of situation offered to the group by the teacher and the behavior of the target participant. The situation offered to the group was subdivided into three parts: preparatory situations; knowledge development situations, and motor development situations.
The observations and coding of behaviors are carried out “at intervals.” This technique is used extensively in research on behavior analysis. The coder observes the teaching situation and a particular student during each interval ( Brunelle et al., 1988 ). It then makes a decision concerning the characteristic of the observed behavior. The 6-s observation interval is followed by a coding interval of 6 s too. A cassette tape recorder is used to regulate the observation and recording intervals. It is recorded for this purpose with the indices “observe” and “code” at the start of each 6-s period. During each coding unit, the observer answered the following questions: What is the type of situation in which the class group finds itself? If the class group is in a learning situation proper, in what form of commitment does the observed student find himself? The abbreviations representing the various categories of behavior have been entered in the spaces which correspond to them. The coder was asked to enter a hyphen instead of the abbreviation when the same categories of behavior follow one another in consecutive intervals ( Brunelle et al., 1988 ).
During the preparatory period, the following behaviors were identified and analyzed:
- Deviant behavior: The student adopts a behavior incompatible with a listening attitude or with the smooth running of the preparatory situations.
- Waiting time: The student is waiting without listening or observing.
- Organized during: The student is involved in a complementary activity that does not represent a contribution to learning (e.g., regaining his place in a line, fetching a ball that has just left the field, replacing a piece of equipment).
During the motor development situations, the following behaviors were identified and analyzed:
- Motor engagement 1: The participant performs the motor activity with such easy that it can be inferred that their actions have little chance to engage in a learning process.
- Motor engagement 2: The participant-despite a certain degree of difficulty, performs the motor activity with sufficient success, which makes it possible to infer that they are in the process of learning.
- Motor engagement 3: The participant performs the motor activity with such difficulty that their efforts have very little chance of being part of a learning process.
2.4. Statistical analysis
Statistical tests were performed using statistical software 26.0 for windows (SPSS, Inc, Chicago, IL, USA). Data are presented in text and tables as means ± standard deviations and in figures as means and standard errors. Once the normal distribution of data was confirmed by the Shapiro-Wilk W -test, parametric tests were performed. Analysis of the results was performed using a mixed 2-way analysis of variance (ANOVA): Groups × Time with repeated measures.
For the learning parameters, the ANOVA took the following form: 2 Groups (Control Group vs. Experimental Group) × 3 Times (T0, T1, and T2).
For the dimensions of motivation, the ANOVA took the following form: 2 Groups (Control Group vs. Experimental Group) × 2 Time (T0 vs. T2).
In instances where the ANOVA showed a significant effect, a Bonferroni post-hoc test was applied in order to compare the experimental data in pairs, otherwise by an independent or paired Student's T -test. Effect sizes were calculated as partial eta-squared η p 2 to estimate the meaningfulness of significant findings, where η p 2 values of 0.01, 0.06, and 0.13 represent small, moderate, and large effect sizes, respectively ( Lakens, 2013 ). All observed differences were considered statistically significant for a probability threshold lower than p < 0.05.
Table 2 shows the results of learning variables during the preparatory and the development learning periods at T0, T1, and T2, in the control group and the experimental group.
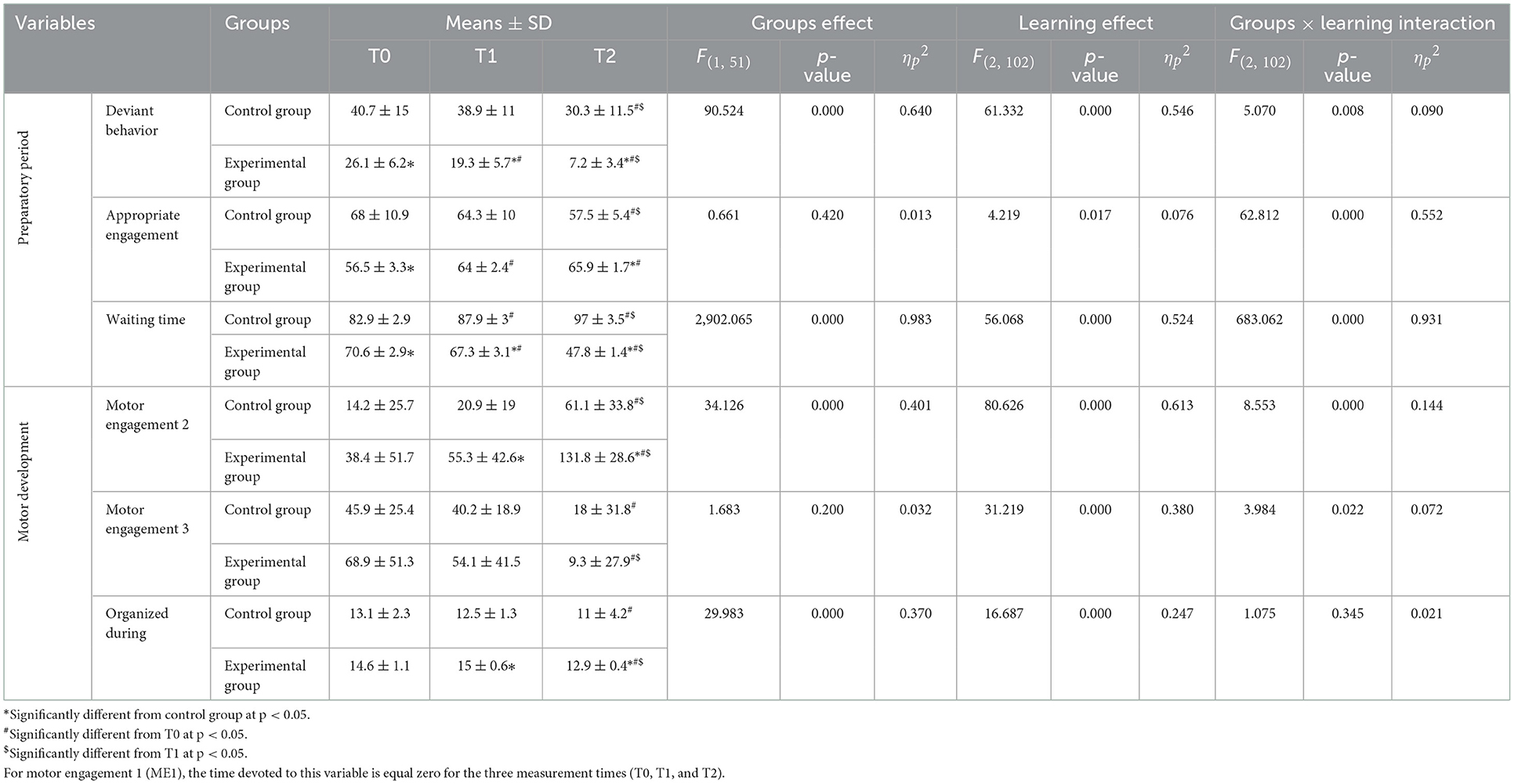
Table 2 . Comparison of learning variables using two teaching methods in physical education.
The analysis of variance of two factors with repeated measures showed a significant effect of group, learning, and group learning interaction for the deviant behavior. The post-hoc test revealed significantly less frequent deviant behaviors in the experimental than in the control group at T0, T1, and T2 (all p < 0.001). Additionally, the deviant behavior decreased significantly at T1 and T2 compared to T0 for both groups (all p < 0.001).
For appropriate engagement, there were no significant group effect, a significant learning effect, and a significant group learning interaction effect. The post-hoc test revealed that compared to T0, Appropriate engagement recorded at T1 and T2 increased significantly ( p = 0.032; p = 0.031, respectively) in the experimental group, whilst it decreased significantly in the control group ( p < 0.001). Additionally, Appropriate engagement was higher in the experimental vs. control group at T1 and T2 (all p < 0.001).
For waiting time, a significant interaction in terms of group effect, learning, and group learning was found. The post-hoc test revealed that waiting time was higher at T1 and T2 vs. T0 (all p < 0.001) in the control group. In addition, waiting time in the experimental group decreased significantly at T1 and T2 vs. T0 (all p < 0.001), with higher values recorded at T2 vs. T1 ( p = 0.025). Additionally, lower values were recorded in the experimental group vs. the control group at the three-time points (all p < 0.001).
For Motor engagement 2, a significant group, learning, and group-learning interaction effect was noted. The post-hoc test revealed that Motor engagement 2 increased significantly in both groups at T1 ( p < 0.0001) and T2 ( p < 0.0001) vs. T0 ( p = 0.045), with significantly higher values recorded in the experimental group at T1 and T2.
Regarding Motor engagement 3, a non-significant group effect was reported. Contrariwise, a significant learning effect and group learning interaction was reported ( Table 1 ). The post-hoc test revealed a significant decrease in the control group and the experimental group at T1 ( p = 0.294) at T2 ( p = 0.294) vs. T0 ( p = 0.0543). In addition, a non-significant difference between the two groups was found.
A significant group and learning effect was noted for the organized during, and a non-significant group learning interaction. For organized during, the paired Student T -test showed a significant decrease in the control group and the experimental group (all p < 0.001). The independent Student T -test revealed a non-significant difference between groups at the three-time points.
Results of the motivational dimensions in the control group and the experimental group recorded at T0 and T2 are presented in Table 3 .
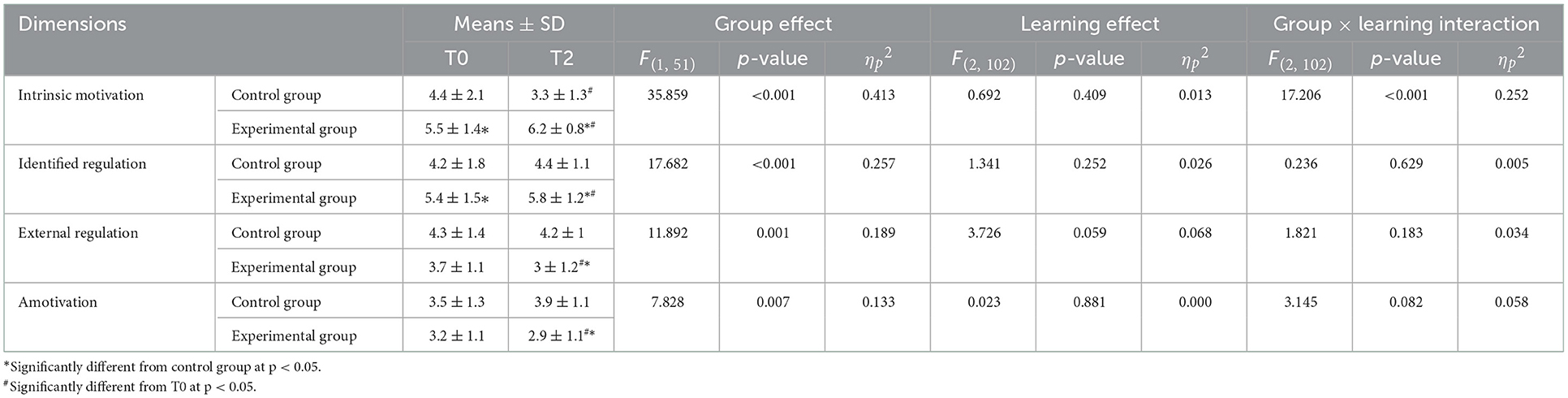
Table 3 . Comparison of the four motivational dimensions in two teaching methods in physical education.
For intrinsic motivation, a significant group effect and group learning interaction and also a non-significant learning effect was found. The post-hoc test indicated that the intrinsic motivation decreased significantly in the control group ( p = 0.029), whilst it increased in the experimental group ( p = 0.04). Additionally, the intrinsic motivation of the experimental group was higher at T0 ( p = 0.026) and T2 ( p < 0.001) compared to that of the control group.
For the identified regulation, a significant group effect, a non-significant learning effect and group learning interaction were reported. The paired Student's T -test revealed that from T0 to T1, the identified motivation increased significantly only in the experimental group ( p = 0.022), while it remained unchanged in the control group. The independent Student's T -test revealed that the identified regulation recorded in the experimental group at T0 ( p = 0.012) and T2 ( p < 0.001) was higher compared to that of the control group.
The external regulation presents a significant group effect. In addition, a non-significant learning effect and group learning interaction were reported. The paired Student's T -test showed that the external regulation decreased significantly in the experimental group ( p = 0.038), whereas it remained unchanged in the control group. Further, the independent Student's T -test revealed that the external regulation recorded at T2 was higher in the control group vs. the experimental group ( p < 0.001).
Relating to amotivation, results showed a significant group effect. Furthermore, a non-significant learning effect and group learning interaction were reported. The paired Student's T -test showed that, from T0 to T2, amotivation decreased significantly in the experimental group ( p = 0.011) and did not change in the control group. The independent Student T -test revealed that amotivation recorded at T2 was lower in the experimental compared to the control group ( p = 0.002).
4. Discussion
The main purpose of this study was to compare the effects of the problem-solving vs. traditional method on motivation and learning during physical education courses. The results revealed that the problem-solving method is more effective than the traditional method in increasing students' motivation and improving their learning. Moreover, the results showed that mean wait times and deviant behaviors decreased using the problem-solving method. Interestingly, the average time spent on appropriate engagement increased using the problem-solving method compared to the traditional method. When using the traditional method, the average wait times increased and, as a result, the time spent on appropriate engagement decreased. Then, following the decrease in deviant behaviors and waiting times, an increase in the time spent warming up was evident (i.e., appropriate engagement). Indeed, there was an improvement in engagement time using the problem-solving method and a decrease using the traditional method. On the other hand, there was a decrease in motor engagement 3 in favor of motor engagement 2. Indeed, it has been shown that the problem-solving method has been used in the learning process and allows for its improvement ( Docktor et al., 2015 ). In addition, it could also produce better quality solutions and has higher scores on conceptual and problem-solving measures. It is also a good method for the learning process to enhance students' academic performance ( Docktor et al., 2015 ; Ali, 2019 ). In contrast, the traditional method limits the ability of teachers to reach and engage all students ( Cook and Artino, 2016 ). Furthermore, it produces passive learning with an understanding of basic knowledge which is characterized by its weakness ( Goldstein, 2016 ). Taken together, it appears that the problem-solving method promotes and improves learning more than the traditional method.
It should be acknowledged that other factors, such as motivation, could influence learning. In this context, our results showed that the method of problem-solving could improve the motivation of the learners. This motivation includes several variables that change depending on the situation, namely the intrinsic motivation that pushes the learner to engage in an activity for the interest and pleasure linked to the practice of the latter ( Komarraju et al., 2009 ; Guiffrida et al., 2013 ; Chedru, 2015 ). The student, therefore, likes to learn through problem-solving and neglects that of the traditional method. These results are concordant with others ( Deci and Ryan, 1985 ; Chedru, 2015 ; Ryan and Deci, 2020 ). Regarding the three forms of extrinsic motivation: first, extrinsic motivation by an identified regulation which manifests itself in a high degree of self-determination where the learner engages in the activity because it is important for him ( Deci and Ryan, 1985 ; Chedru, 2015 ). This explains the significant difference between the two groups. Then, the motivation by external regulation which is characterized by a low degree of self-determination such as the behavior of the learner is manipulated by external circumstances such as obtaining rewards or the removal of sanctions ( Deci and Ryan, 1985 ; Chedru, 2015 ). For this, the means of this variable decreased for the experimental group which is intrinsically motivated. He does not need any reward to work and is not afraid of punishment because he is self-confident. Third, amotivation is at the opposite end of the self-determination continuum. Unmotivated students are the most likely to feel negative emotions ( Ratelle et al., 2007 ; David, 2010 ), to have low self-esteem ( Deci and Ryan, 1995 ), and who attempts to abandon their studies ( Vallerand et al., 1997 ; Blanchard et al., 2005 ). So, more students are motivated by external regulation or demotivated, less interest they show and less effort they make, and more likely they are to fail ( Grolnick et al., 1991 ; Miserandino, 1996 ; Guay et al., 2000 ; Blanchard et al., 2005 ).
It is worth noting that there is a close link between motivation and learning ( Bessa et al., 2021 ; Rossa et al., 2021 ). Indeed, when the learner's motivation is high, so will his learning. However, all this depends on the method used ( Norboev, 2021 ). For example, the method of problem-solving increase motivation more than the traditional method, as evidenced by several researchers ( Parish and Treasure, 2003 ; Artino and Stephens, 2009 ; Kim and Frick, 2011 ; Lemos and Veríssimo, 2014 ).
Given the effectiveness of the problem-solving method in improving students' learning and motivation, it should be used during physical education teaching. This could be achieved through the organization of comprehensive training programs, seminars, and workshops for teachers so to master and subsequently be able to use the problem-solving method during physical education lessons.
Despite its novelty, the present study suffers from a few limitations that should be acknowledged. First, a future study, consisting of a group taught using the mixed method would preferable so to better elucidate the true impact of this teaching and learning method. Second, no gender and/or age group comparisons were performed. This issue should be addressed in future investigations. Finally, the number of participants is limited. This may be due to working in a secondary school where the number of students in a class is limited to 30 students. Additionally, the number of participants fell to 53 after excluding certain students (exempted, absent for a session, exercising in civil clubs or member of the school association). Therefore, to account for classes of finite size, a cluster-based trial would be beneficial in the future. Moreover, future studies investigating the effect of the active method in reducing some behaviors (e.g., disruptive behaviors) and for the improvement of pupils' attention are warranted.
5. Conclusion
There was an improvement in student learning in favor of the problem-solving method. Additionally, we found that the motivation of learners who were taught using the problem-solving method was better than that of learners who were educated by the traditional method.
Data availability statement
The original contributions presented in the study are included in the article/supplementary material, further inquiries can be directed to the corresponding author.
Ethics statement
University Research Ethics Board approval was obtained before recruiting participants who were subsequently informed of the nature, objective, methodology, and constraints. Teacher, school director, parental/guardian, and child informed consent was obtained prior to participation in the study. In addition, exclusion criteria included; the practice of handball activity in civil/competitive/amateur clubs or in the high school sports association. Written informed consent to participate in this study was provided by the participants' legal guardian/next of kin.
Author contributions
All authors listed have made a substantial, direct, and intellectual contribution to the work and approved it for publication.
Acknowledgments
Special thanks for all students and physical education teaching staff from the 15 November 1955 Secondary School, who generously shared their time, experience, and materials for the proposes of this study.
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
The reviewer MJ declared a shared affiliation, with no collaboration, with the authors GE, NS, LM, and KT to the handling editor at the time of review.
Publisher's note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
Adunola, O., Ed, B., and Adeniran, A. (2012). The Impact of Teachers' Teaching Methods on the Academic Performance of Primary School Pupils . Ogun: Ego Booster Books.
Google Scholar
Ali, S. S. (2019). Problem based learning: A student-centered approach. Engl. Lang. Teach . 12, 73. doi: 10.5539/elt.v12n5p73
CrossRef Full Text | Google Scholar
Ang, S. C., and Penney, D. (2013). Promoting social and emotional learning outcomes in physical education: Insights from a school-based research project in Singapore. Asia-Pac. J. Health Sport Phys. Educ . 4, 267–286. doi: 10.1080/18377122.2013.836768
Artino, A. R., and Stephens, J. M. (2009). Academic motivation and self-regulation: A comparative analysis of undergraduate and graduate students learning online. Internet. High. Educ. 12, 146–151. doi: 10.1016/j.iheduc.2009.02.001
Arvind, K., and Kusum, G. (2017). Teaching approaches, methods and strategy. Sch. Res. J. Interdiscip. Stud. 4, 6692–6697. doi: 10.21922/srjis.v4i36.10014
Ayeni, A. J. (2011). Teachers' professional development and quality assurance in Nigerian secondary schools. World J. Educ. 1, 143. doi: 10.5430/wje.v1n2p143
Bessa, C., Hastie, P., Rosado, A., and Mesquita, I. (2021). Sport education and traditional teaching: Influence on students' empowerment and self-confidence in high school physical education classes. Sustainability 13, 578. doi: 10.3390/su13020578
Bi, M., Zhao, Z., Yang, J., and Wang, Y. (2019). Comparison of case-based learning and traditional method in teaching postgraduate students of medical oncology. Med. Teach . 4, 1124–1128. doi: 10.1080/0142159X.2019.1617414
PubMed Abstract | CrossRef Full Text | Google Scholar
Blanchard, C., Pelletier, L., Otis, N., and Sharp, E. (2005). Role of self-determination and academic ability in predicting school absences and intention to drop out. J. Educ. Sci. 30, 105–123. doi: 10.7202/011772ar
Blázquez, D. (2016).Métodos de enseñanza en Educación Física. Enfoques innovadores para la enseñanza de competencias . Barcelona: Inde Publisher.
Brunelle, J., Drouin, D., Godbout, P., and Tousignant, M. (1988). Supervision of physical activity intervention. Montreal, Canada: G. Morin, Dl. Open J. Soc. Sci. 8.
Bunker, D., and Thorpe, R. (1982). A model for the teaching of games in secondary schools. Bull. Phys. Educ . 18, 5–8.
Casey, A. (2014). Models-based practice: Great white hope or white elephant? Phys. Educ. Sport Pedagog. 19, 18–34. doi: 10.1080/17408989.2012.726977
Casey, A., MacPhail, A., Larsson, H., and Quennerstedt, M. (2021). Between hope and happening: Problematizing the M and the P in models-based practice. Phys. Educ. Sport Pedagog. 26, 111–122. doi: 10.1080/17408989.2020.1789576
Chedru, M. (2015). Impact of motivation and learning styles on the academic performance of engineering students. J. Educ. Sci. 41, 457–482. doi: 10.7202/1035313ar
Cook, D. A., and Artino, A. R. (2016). Motivation to learn: An overview of contemporary theories. J. Med. Educ . 50, 997–1014. doi: 10.1111/medu.13074
Cronbach, L. J. (1951). Coefficient alpha and the internal structure of tests. Psychometrika. J. 16, 297–334. doi: 10.1007/BF02310555
Cunningham, J., and Sood, K. (2018). How effective are working memory training interventions at improving maths in schools: a study into the efficacy of working memory training in children aged 9 and 10 in a junior school?. Education 46, 174–187. doi: 10.1080/03004279.2016.1210192
Darling-Hammond, L., Flook, L., Cook-Harvey, C., Barron, B., and Osher, D. (2020). Implications for educational practice of the science of learning and development. Appl. Dev. Sci. 24, 97–140. doi: 10.1080/10888691.2018.1537791
David, A. (2010). Examining the relationship of personality and burnout in college students: The role of academic motivation. E. M. E. Rev. 1, 90–104.
Deci, E., and Ryan, R. (1985). Intrinsic motivation and self-determination in human behavior. Perspect. Soc. Psychol . 2271, 7. doi: 10.1007/978-1-4899-2271-7
Deci, E. L., and Ryan, R. M. (1995). “Human autonomy,” in Efficacy, Agency, and Self-Esteem (Boston, MA: Springer).
Dickinson, B. L., Lackey, W., Sheakley, M., Miller, L., Jevert, S., and Shattuck, B. (2018). Involving a real patient in the design and implementation of case-based learning to engage learners. Adv. Physiol. Educ. 42, 118–122. doi: 10.1152/advan.00174.2017
Docktor, J. L., Strand, N. E., Mestre, J. P., and Ross, B. H. (2015). Conceptual problem solving in high school. Phys. Rev. ST Phys. Educ. Res. 11, 20106. doi: 10.1103/PhysRevSTPER.11.020106
Dyson, B., and Grineski, S. (2001). Using cooperative learning structures in physical education. J. Phys. Educ. Recreat. Dance. 72, 28–31. doi: 10.1080/07303084.2001.10605831
Ergül, N. R., and Kargin, E. K. (2014). The effect of project based learning on students' science success. Procedia. Soc. Behav. Sci. 136, 537–541. doi: 10.1016/j.sbspro.2014.05.371
Fenouillet, F. (2012). Les théories de la motivation . Psycho Sup : Dunod.
Fidan, M., and Tuncel, M. (2019). Integrating augmented reality into problem based learning: The effects on learning achievement and attitude in physics education. Comput. Educ .142, 103635. doi: 10.1016/j.compedu.2019.103635
Garrett, T. (2008). Student-centered and teacher-centered classroom management: A case study of three elementary teachers. J. Classr. Interact. 43, 34–47.
Goldstein, O. A. (2016). Project-based learning approach to teaching physics for pre-service elementary school teacher education students. Bevins S, éditeur. Cogent. Educ. 3, 1200833. doi: 10.1080/2331186X.2016.1200833
Gordon, B., and Doyle, S. (2015). Teaching personal and social responsibility and transfer of learning: Opportunities and challenges for teachers and coaches. J. Teach. Phys. Educ. 34, 152–161. doi: 10.1123/jtpe.2013-0184
Grolnick, W. S., Ryan, R. M., and Deci, E. L. (1991). Inner resources for school achievement: Motivational mediators of children's perceptions of their parents. J. Educ. Psychol . 83, 508–517. doi: 10.1037/0022-0663.83.4.508
Guay, F., Vallerand, R. J., and Blanchard, C. (2000). On the assessment of situational intrinsic and extrinsic motivation: The Situational Motivation Scale (SIMS). Motiv. Emot . 24, 175–213. doi: 10.1023/A:1005614228250
Guiffrida, D., Lynch, M., Wall, A., and Abel, D. (2013). Do reasons for attending college affect academic outcomes? A test of a motivational model from a self-determination theory perspective. J. Coll. Stud. Dev. 54, 121–139. doi: 10.1353/csd.2013.0019
Hastie, P. A., and Casey, A. (2014). Fidelity in models-based practice research in sport pedagogy: A guide for future investigations. J. Teach. Phys. Educ . 33, 422–431. doi: 10.1123/jtpe.2013-0141
Hu, W. (2010). Creative scientific problem finding and its developmental trend. Creat. Res. J. 22, 46–52. doi: 10.1080/10400410903579551
Ilkiw, J. E., Nelson, R. W., Watson, J. L., Conley, A. J., Raybould, H. E., Chigerwe, M., et al. (2017). Curricular revision and reform: The process, what was important, and lessons learned. J. Vet. Med. Educ . 44, 480–489. doi: 10.3138/jvme.0316-068R
Johnson, A. P. (2010). Making Connections in Elementary and Middle School Social Studies. 2nd Edn. London: SAGE.
Johnson, D. W., Johnson, R. T., and Smith, K. A. (1998). Active Learning: Cooperation in the College Classroom. 2nd Edn. Edina, MN: Interaction Press.
Kim, K. J., and Frick, T. (2011). Changes in student motivation during online learning. J. Educ. Comput. 44, 23. doi: 10.2190/EC.44.1.a
Kolesnikova, I. (2016). Combined teaching method: An experimental study. World J. Educ. 6, 51–59. doi: 10.5430/wje.v6n6p51
Komarraju, M., Karau, S. J., and Schmeck, R. R. (2009). Role of the Big Five personality traits in predicting college students' academic motivation and achievement. Learn. Individ. Differ. 19, 47–52. doi: 10.1016/j.lindif.2008.07.001
Lakens, D. (2013). Calculating and reporting effect sizes to facilitate cumulative science: A practical primer for t -tests and ANOVAs. Front. Psychol . 4, 863. doi: 10.3389/fpsyg.2013.00863
Lemos, M. S., and Veríssimo, L. (2014). The relationships between intrinsic motivation, extrinsic motivation, and achievement, along elementary school. Procedia. Soc. Behav. Sci . 112, 930–938. doi: 10.1016/j.sbspro.2014.01.1251
Leo, M. F., Mouratidis, A., Pulido, J. J., López-Gajardo, M. A., and Sánchez-Oliva, D. (2022). Perceived teachers' behavior and students' engagement in physical education: the mediating role of basic psychological needs and self-determined motivation. Phys. Educ. Sport Pedagogy. 27, 59–76. doi: 10.1080/17408989.2020.1850667
Lonsdale, C., Sabiston, C., Taylor, I., and Ntoumanis, N. (2011). Measuring student motivation for physical education: Examining the psychometric properties of the Perceived Locus of Causality Questionnaire and the Situational Motivation Scale. Psychol. Sport. Exerc . 12, 284–292. doi: 10.1016/j.psychsport.2010.11.003
Luo, Y. J. (2019). The influence of problem-based learning on learning effectiveness in students' of varying learning abilities within physical education. Innov. Educ. Teach. Int. 56, 3–13. doi: 10.1080/14703297.2017.1389288
Maddeh, T., Amamou, S., Desbiens, J., and françois Souissi, N. (2020). The management of disruptive behavior of secondary school students by Tunisian trainee teachers in physical education: Effects of a training program in the prevention and management of indiscipline. J. Educ. Fac . 4, 323–344. doi: 10.34056/aujef.674931
Manninen, M., and Campbell, S. (2021). The effect of the sport education model on basic needs, intrinsic motivation and prosocial attitudes: A systematic review and multilevel meta-Analysis. Eur. Phys. Educ. Rev . 28, 76–99. doi: 10.1177/1356336X211017938
Menendez, J. I., and Fernandez-Rio, J. (2017). Hybridising sport education and teaching for personal and social responsibility to include students with disabilities. Eur. J. Spec. Needs Educ. 32, 508–524 doi: 10.1080/08856257.2016.1267943
Metzler, M. (2017). Instructional Models in Physical Education . London: Routledge.
Miserandino, M. (1996). Children who do well in school: Individual differences in perceived competence and autonomy in above-average children. J. Educ. Psychol . 88, 203–214. doi: 10.1037/0022-0663.88.2.203
Norboev, N. N. (2021). Theoretical aspects of the influence of motivation on increasing the efficiency of physical education. Curr. Res. J. Pedagog . 2, 247–252. doi: 10.37547/pedagogics-crjp-02-10-44
Novak, D. J. (2010). Learning, creating, and using knowledge: Concept maps as facilitative tools in schools and corporations. J. E-Learn. Knowl. Soc . 6, 21–30. doi: 10.20368/1971-8829/441
Parish, L. E., and Treasure, D. C. (2003). Physical activity and situational motivation in physical education: Influence of the motivational climate and perceived ability. Res. Q. Exerc. Sport. 74, 173. doi: 10.1080/02701367.2003.10609079
Pérez-Jorge, D., González-Dorta, D., Del Carmen Rodríguez-Jiménez, D., and Fariña-Hernández, L. (2021). Problem-solving teacher training, the effect of the ProyectaMates Programme in Tenerife. International Educ . 49, 777–7791. doi: 10.1080/03004279.2020.1786427
Perrenoud, P. (2003). Qu'est-ce qu'apprendre. Enfances Psy . 4, 9–17. doi: 10.3917/ep.024.0009
Pohan, A., Asmin, A., and Menanti, A. (2020). The effect of problem based learning and learning motivation of mathematical problem solving skills of class 5 students at SDN 0407 Mondang. BirLE . 3, 531–539. doi: 10.33258/birle.v3i1.850
Puigarnau, S., Camerino, O., Castañer, M., Prat, Q., and Anguera, M. T. (2016). El apoyo a la autonomía en practicantes de centros deportivos y de fitness para aumentar su motivación. Rev. Int. de Cienc. del deporte. 43, 48–64. doi: 10.5232/ricyde2016.04303
Ratelle, C. F., Guay, F., Vallerand, R. J., Larose, S., and Senécal, C. (2007). Autonomous, controlled, and amotivated types of academic motivation: A person-oriented analysis. J. Educ. Psychol. 99, 734–746. doi: 10.1037/0022-0663.99.4.734
Rivera-Pérez, S., Fernandez-Rio, J., and Gallego, D. I. (2020). Effects of an 8-week cooperative learning intervention on physical education students' task and self-approach goals, and Emotional Intelligence. Int. J. Environ. Res. Public Health . 18, 61. doi: 10.3390/ijerph18010061
Rolland, V. (2009). Motivation in the School Context . 5th Edn . Belguim: De Boeck Superior, 218.
Rossa, R., Maulidiah, R. H., and Aryni, Y. (2021). The effect of problem solving technique and motivation toward students' writing skills. J. Pena. Edukasi. 8, 43–54. doi: 10.54314/jpe.v8i1.654
Ryan, R., and Deci, E. (2020). Intrinsic and extrinsic motivation from a self-determination theory perspective: Definitions, theory, practices, and future directions. Contemp. Educ. Psychol . 61, 101860. doi: 10.1016/j.cedpsych.2020.101860
Siedentop, D., Hastie, P. A., and Van Der Mars, H. (2011). Complete Guide to Sport Education, 2nd Edn. Champaign, IL: Human Kinetics.
Skinner, B. F. (1985). Cognitive science and behaviourism. Br. J. Psychol. 76, 291–301. doi: 10.1111/j.2044-8295.1985.tb01953.x
Standage, M., Gillison, F. B., Ntoumanis, N., and Treasure, D. C. (2012). Predicting students' physical activity and health-related well-being: a prospective cross-domain investigation of motivation across school physical education and exercise settings. J. Sport. Exerc. Psychol . 34, 37–60. doi: 10.1123/jsep.34.1.37
Tebabal, A., and Kahssay, G. (2011). The effects of student-centered approach in improving students' graphical interpretation skills and conceptual understanding of kinematical motion. Lat. Am. J. Phys. Educ. 5, 9.
Vallerand, R. J., Fbrtier, M. S., and Guay, F. (1997). Self-determination and persistence in a real-life setting toward a motivational model of high school dropout. J. Pers. Soc. Psychol. 2, 1161–1176. doi: 10.1037/0022-3514.72.5.1161
Ward, P., Mitchell, M. F., van der Mars, H., and Lawson, H. A. (2021). Chapter 3: PK+12 School physical education: conditions, lessons learned, and future directions. J. Teach. Phys. Educ. 40, 363–371. doi: 10.1123/jtpe.2020-0241
Keywords: problem-solving method, traditional method, motivation, learning, students
Citation: Ezeddine G, Souissi N, Masmoudi L, Trabelsi K, Puce L, Clark CCT, Bragazzi NL and Mrayah M (2023) The problem-solving method: Efficacy for learning and motivation in the field of physical education. Front. Psychol. 13:1041252. doi: 10.3389/fpsyg.2022.1041252
Received: 10 September 2022; Accepted: 15 December 2022; Published: 25 January 2023.
Reviewed by:
Copyright © 2023 Ezeddine, Souissi, Masmoudi, Trabelsi, Puce, Clark, Bragazzi and Mrayah. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY) . The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Want to create or adapt books like this? Learn more about how Pressbooks supports open publishing practices.
5 Teaching Mathematics Through Problem Solving
Janet Stramel

In his book “How to Solve It,” George Pólya (1945) said, “One of the most important tasks of the teacher is to help his students. This task is not quite easy; it demands time, practice, devotion, and sound principles. The student should acquire as much experience of independent work as possible. But if he is left alone with his problem without any help, he may make no progress at all. If the teacher helps too much, nothing is left to the student. The teacher should help, but not too much and not too little, so that the student shall have a reasonable share of the work.” (page 1)
What is a problem in mathematics? A problem is “any task or activity for which the students have no prescribed or memorized rules or methods, nor is there a perception by students that there is a specific ‘correct’ solution method” (Hiebert, et. al., 1997). Problem solving in mathematics is one of the most important topics to teach; learning to problem solve helps students develop a sense of solving real-life problems and apply mathematics to real world situations. It is also used for a deeper understanding of mathematical concepts. Learning “math facts” is not enough; students must also learn how to use these facts to develop their thinking skills.
According to NCTM (2010), the term “problem solving” refers to mathematical tasks that have the potential to provide intellectual challenges for enhancing students’ mathematical understanding and development. When you first hear “problem solving,” what do you think about? Story problems or word problems? Story problems may be limited to and not “problematic” enough. For example, you may ask students to find the area of a rectangle, given the length and width. This type of problem is an exercise in computation and can be completed mindlessly without understanding the concept of area. Worthwhile problems includes problems that are truly problematic and have the potential to provide contexts for students’ mathematical development.
There are three ways to solve problems: teaching for problem solving, teaching about problem solving, and teaching through problem solving.
Teaching for problem solving begins with learning a skill. For example, students are learning how to multiply a two-digit number by a one-digit number, and the story problems you select are multiplication problems. Be sure when you are teaching for problem solving, you select or develop tasks that can promote the development of mathematical understanding.
Teaching about problem solving begins with suggested strategies to solve a problem. For example, “draw a picture,” “make a table,” etc. You may see posters in teachers’ classrooms of the “Problem Solving Method” such as: 1) Read the problem, 2) Devise a plan, 3) Solve the problem, and 4) Check your work. There is little or no evidence that students’ problem-solving abilities are improved when teaching about problem solving. Students will see a word problem as a separate endeavor and focus on the steps to follow rather than the mathematics. In addition, students will tend to use trial and error instead of focusing on sense making.
Teaching through problem solving focuses students’ attention on ideas and sense making and develops mathematical practices. Teaching through problem solving also develops a student’s confidence and builds on their strengths. It allows for collaboration among students and engages students in their own learning.
Consider the following worthwhile-problem criteria developed by Lappan and Phillips (1998):
- The problem has important, useful mathematics embedded in it.
- The problem requires high-level thinking and problem solving.
- The problem contributes to the conceptual development of students.
- The problem creates an opportunity for the teacher to assess what his or her students are learning and where they are experiencing difficulty.
- The problem can be approached by students in multiple ways using different solution strategies.
- The problem has various solutions or allows different decisions or positions to be taken and defended.
- The problem encourages student engagement and discourse.
- The problem connects to other important mathematical ideas.
- The problem promotes the skillful use of mathematics.
- The problem provides an opportunity to practice important skills.
Of course, not every problem will include all of the above. Sometimes, you will choose a problem because your students need an opportunity to practice a certain skill.
Key features of a good mathematics problem includes:
- It must begin where the students are mathematically.
- The feature of the problem must be the mathematics that students are to learn.
- It must require justifications and explanations for both answers and methods of solving.

Problem solving is not a neat and orderly process. Think about needlework. On the front side, it is neat and perfect and pretty.

But look at the b ack.
It is messy and full of knots and loops. Problem solving in mathematics is also like this and we need to help our students be “messy” with problem solving; they need to go through those knots and loops and learn how to solve problems with the teacher’s guidance.
When you teach through problem solving , your students are focused on ideas and sense-making and they develop confidence in mathematics!
Mathematics Tasks and Activities that Promote Teaching through Problem Solving

Choosing the Right Task
Selecting activities and/or tasks is the most significant decision teachers make that will affect students’ learning. Consider the following questions:
- Teachers must do the activity first. What is problematic about the activity? What will you need to do BEFORE the activity and AFTER the activity? Additionally, think how your students would do the activity.
- What mathematical ideas will the activity develop? Are there connections to other related mathematics topics, or other content areas?
- Can the activity accomplish your learning objective/goals?

Low Floor High Ceiling Tasks
By definition, a “ low floor/high ceiling task ” is a mathematical activity where everyone in the group can begin and then work on at their own level of engagement. Low Floor High Ceiling Tasks are activities that everyone can begin and work on based on their own level, and have many possibilities for students to do more challenging mathematics. One gauge of knowing whether an activity is a Low Floor High Ceiling Task is when the work on the problems becomes more important than the answer itself, and leads to rich mathematical discourse [Hover: ways of representing, thinking, talking, agreeing, and disagreeing; the way ideas are exchanged and what the ideas entail; and as being shaped by the tasks in which students engage as well as by the nature of the learning environment].
The strengths of using Low Floor High Ceiling Tasks:
- Allows students to show what they can do, not what they can’t.
- Provides differentiation to all students.
- Promotes a positive classroom environment.
- Advances a growth mindset in students
- Aligns with the Standards for Mathematical Practice
Examples of some Low Floor High Ceiling Tasks can be found at the following sites:
- YouCubed – under grades choose Low Floor High Ceiling
- NRICH Creating a Low Threshold High Ceiling Classroom
- Inside Mathematics Problems of the Month
Math in 3-Acts
Math in 3-Acts was developed by Dan Meyer to spark an interest in and engage students in thought-provoking mathematical inquiry. Math in 3-Acts is a whole-group mathematics task consisting of three distinct parts:
Act One is about noticing and wondering. The teacher shares with students an image, video, or other situation that is engaging and perplexing. Students then generate questions about the situation.
In Act Two , the teacher offers some information for the students to use as they find the solutions to the problem.
Act Three is the “reveal.” Students share their thinking as well as their solutions.
“Math in 3 Acts” is a fun way to engage your students, there is a low entry point that gives students confidence, there are multiple paths to a solution, and it encourages students to work in groups to solve the problem. Some examples of Math in 3-Acts can be found at the following websites:
- Dan Meyer’s Three-Act Math Tasks
- Graham Fletcher3-Act Tasks ]
- Math in 3-Acts: Real World Math Problems to Make Math Contextual, Visual and Concrete
Number Talks
Number talks are brief, 5-15 minute discussions that focus on student solutions for a mental math computation problem. Students share their different mental math processes aloud while the teacher records their thinking visually on a chart or board. In addition, students learn from each other’s strategies as they question, critique, or build on the strategies that are shared.. To use a “number talk,” you would include the following steps:
- The teacher presents a problem for students to solve mentally.
- Provide adequate “ wait time .”
- The teacher calls on a students and asks, “What were you thinking?” and “Explain your thinking.”
- For each student who volunteers to share their strategy, write their thinking on the board. Make sure to accurately record their thinking; do not correct their responses.
- Invite students to question each other about their strategies, compare and contrast the strategies, and ask for clarification about strategies that are confusing.
“Number Talks” can be used as an introduction, a warm up to a lesson, or an extension. Some examples of Number Talks can be found at the following websites:
- Inside Mathematics Number Talks
- Number Talks Build Numerical Reasoning

Saying “This is Easy”
“This is easy.” Three little words that can have a big impact on students. What may be “easy” for one person, may be more “difficult” for someone else. And saying “this is easy” defeats the purpose of a growth mindset classroom, where students are comfortable making mistakes.
When the teacher says, “this is easy,” students may think,
- “Everyone else understands and I don’t. I can’t do this!”
- Students may just give up and surrender the mathematics to their classmates.
- Students may shut down.
Instead, you and your students could say the following:
- “I think I can do this.”
- “I have an idea I want to try.”
- “I’ve seen this kind of problem before.”
Tracy Zager wrote a short article, “This is easy”: The Little Phrase That Causes Big Problems” that can give you more information. Read Tracy Zager’s article here.
Using “Worksheets”
Do you want your students to memorize concepts, or do you want them to understand and apply the mathematics for different situations?
What is a “worksheet” in mathematics? It is a paper and pencil assignment when no other materials are used. A worksheet does not allow your students to use hands-on materials/manipulatives [Hover: physical objects that are used as teaching tools to engage students in the hands-on learning of mathematics]; and worksheets are many times “naked number” with no context. And a worksheet should not be used to enhance a hands-on activity.
Students need time to explore and manipulate materials in order to learn the mathematics concept. Worksheets are just a test of rote memory. Students need to develop those higher-order thinking skills, and worksheets will not allow them to do that.
One productive belief from the NCTM publication, Principles to Action (2014), states, “Students at all grade levels can benefit from the use of physical and virtual manipulative materials to provide visual models of a range of mathematical ideas.”
You may need an “activity sheet,” a “graphic organizer,” etc. as you plan your mathematics activities/lessons, but be sure to include hands-on manipulatives. Using manipulatives can
- Provide your students a bridge between the concrete and abstract
- Serve as models that support students’ thinking
- Provide another representation
- Support student engagement
- Give students ownership of their own learning.
Adapted from “ The Top 5 Reasons for Using Manipulatives in the Classroom ”.
any task or activity for which the students have no prescribed or memorized rules or methods, nor is there a perception by students that there is a specific ‘correct’ solution method
should be intriguing and contain a level of challenge that invites speculation and hard work, and directs students to investigate important mathematical ideas and ways of thinking toward the learning
involves teaching a skill so that a student can later solve a story problem
when we teach students how to problem solve
teaching mathematics content through real contexts, problems, situations, and models
a mathematical activity where everyone in the group can begin and then work on at their own level of engagement
20 seconds to 2 minutes for students to make sense of questions
Mathematics Methods for Early Childhood Copyright © 2021 by Janet Stramel is licensed under a Creative Commons Attribution 4.0 International License , except where otherwise noted.
Share This Book
Problem Solving Method Of Teaching

| Element | Synthesis | Example |
|---|---|---|
| Active Learning | Teaching through problem-solving allows for active learning. | Children understand the theory better by getting involved in real-world situations |
| Practice | Continuous practice is integral to problem-solving teaching. | Each new skill or concept is practiced after being learned in class. |
| Relevance | Problem-solving techniques make learning more relevant. | Real-world examples related to the topic are presented. |
| Incremental Learning | Each new topic builds on previous lessons. | Relating new problems to ones solved in previous sessions. |
| Overcome Challenges | Enhances ability to overcome real-world situations. | Children understand the application of skills learned. |
| Variety | Problem-solving allows flexibility in teaching methods. | Problems can be practical, conceptual, or theoretical. |
| Critical Thinking | Improves children's critical thinking skills. | Adding alternative paths to a solution. |
| Confidence | Boosts children's confidence in handling problems. | Children feel empowered after successfully solving a problem. |
| Adaptability | Increases adaptability to new learning situations. | Children can apply learned strategies to new problems. |
| Engagement | Problem-solving increases engagement and interest. | Children find solving real-world examples interesting. |
The problem-solving method of teaching is the learning method that allows children to learn by doing. This is because they are given examples and real-world situations so that the theory behind it can be understood better, as well as practice with each new concept or skill taught on top of what was previously learned in class before moving onto another topic at hand.
What is your preferred problem-solving technique?
Answers : - I like to brainstorm and see what works for me - I enjoy the trial and error method - I am a linear thinker
Share it with me by commenting.
For example, while solving a problem, the child may encounter terms he has not studied yet. These will further help him understand their use in context while developing his vocabulary. At the same time, being able to practice math concepts by tapping into daily activities helps an individual retain these skills better.
One way this type of teaching is applied for younger students particularly is through games played during lessons. By allowing them to become comfortable with the concepts taught through these games, they can put their knowledge into use later on. This is done by developing thinking processes that precede an action or behavior. These games can be used by teachers for different subjects including science and language.
For younger students still, the method of teaching using real-life examples helps them understand better. Through this, it becomes easier for them to relate what they learned in school with terms used outside of school settings so that the information sticks better than if all they were given were theoretical definitions. For instance, instead of just studying photosynthesis as part of biology lessons, children are asked to imagine plants growing inside a dark room because there is no sunlight present. When questioned about the plants, children will be able to recall photosynthesis more easily because they were able to see its importance in real life.
Despite being given specific examples, the act of solving problems helps students think for themselves. They learn how to approach situations and predict outcomes based on what they already know about concepts or ideas taught in class including the use of various skills they have acquired over time. These include problem-solving strategies like using drawings when describing a solution or asking advice if they are stuck to unlock solutions that would otherwise go beyond their reach.
Teachers need to point out in advance which method will be used for any particular lesson before having children engage with it. By doing this, individuals can prepare themselves mentally for what is to come. This is especially true for students who have difficulty with a particular subject. In these cases, the teacher can help them get started by providing a worked example for reference or breaking the problem down into manageable chunks that are easier to digest.
JIT (Just-in-Time): A Comprehensive Examination of its Strategic Impact
The Wisdom of Jefferson: Moving, Doing, Thinking
Root Cause Tree Analysis: Insights to Forensic Decision Making
Hazard Analysis: A Comprehensive Approach for Risk Evaluation
Ultimately, the goal of teaching using a problem-solving method is to give children the opportunity to think for themselves and to be able to do so in different contexts. Doing this helps foster independent learners who can utilize the skills they acquired in school for future endeavors.
The problem-solving method of teaching allows children to learn by doing. This is because they are given examples and real-world situations so that the theory behind it can be understood better, as practice with each new concept or skill taught on top of what was previously learned in class before moving onto another topic at hand.
One way this type of teaching is applied for younger students particularly is through games played during lessons. By allowing them to become comfortable with the concepts taught through these games, they are able to put their knowledge into use later on. This is done by developing thinking processes that precede an action or behavior. These games can be used by teachers for different subjects including science and language.
For instance, a teacher may ask students to imagine they are plants in a dark room because there is no sunlight present. When questioned about the plants, children will be able to recall photosynthesis more easily because they were able to see its importance in real life.
It is important for teachers to point out in advance which method will be used for any particular lesson before having children engage with it. By doing this, individuals can prepare themselves mentally for what is to come. This is especially true for students who have difficulty with a particular subject. In these cases, the teacher can help them get started by providing a worked example for reference or breaking the problem down into manageable chunks that are easier to digest.
lesson before having children engage with it. By doing this, individuals can prepare themselves mentally for what is to come. This is especially true for students who have difficulty with a particular subject. In these cases, the teacher can help them get started by providing a worked example for reference or breaking the problem down into manageable chunks that are easier to digest.
The teacher should have a few different ways to solve the problem.
For example, the teacher can provide a worked example for reference or break down the problem into chunks that are easier to digest.
The goal of teaching using a problem-solving method is to give children the opportunity to think for themselves and to be able to do so in different contexts. Successful problem solving allows children to become comfortable with concepts taught through games that develop thinking processes that precede an action or behavior.
Introduce the problem
The problem solving method of teaching is a popular approach to learning that allows students to understand new concepts by doing. This approach provides students with examples and real-world situations, so they can see how the theory behind a concept or skill works in practice. In addition, students are given practice with each new concept or skill taught, before moving on to the next topic. This helps them learn and retain the information better.
Explain why the problem solving method of teaching is effective.
The problem solving method of teaching is effective because it allows students to learn by doing. This means they can see how the theory behind a concept or skill works in practice, which helps them understand and remember the information better. This would not be possible if they are only told about the new concept or skill, or read a textbook to learn on their own. Since students can see how the theory works in practice through examples and real-world situations, the information is easier for them to understand.
List some advantages of using the problem solving method of teaching.
Some advantages of using the problem solving method of teaching are that it helps students retain information better since they are able to practice with each new concept or skill taught until they master it before moving on to another topic. This also allows them to learn by doing so they will have hands-on experience with facts which helps them remember important facts faster rather than just hearing about it or reading about it on their own. Furthermore, this teaching method is beneficial for students of all ages and can be adapted to different subjects making it an approach that is versatile and easily used in a classroom setting. Lastly, the problem solving method of teaching presents new information in a way that is easy to understand so students are not overwhelmed with complex material.
The problem solving method of teaching is an effective way for students to learn new concepts and skills. By providing them with examples and real-world situations, they can see how the theory behind a concept or skill works in practice. In addition, students are given practice with each new concept or skill taught, before moving on to the next topic. This them learn and retain the information better.
What has been your experience with adopting a problem-solving teaching method?
How do you feel the usefulness of your lesson plans changed since adopting this method?
What was one of your most successful attempts in using this technique to teach students, and why do you believe it was so successful?
Were there any obstacles when trying to incorporate this technique into your class?
Did it take a while for all students to get used to the new type of teaching style before they felt comfortable enough to participate in discussions and ask questions about their newly acquired knowledge?
What are your thoughts on this method?
“I have had the opportunity to work in several districts, including one where they used problem solving for all subjects. I never looked back after that experience--it was exciting and motivating for students and teachers alike."
"The problem solving method of teaching is great because it makes my subject matter more interesting with hands-on activities."

What is the role of educators in facilitating problem-solving method of teaching?
Role of Educators in Facilitating Problem-Solving Understanding the Problem-Solving Method The problem-solving method of teaching encourages students to actively engage their critical thinking skills to analyze and seek solutions to real-world problems. As such, educators play a crucial part in facilitating this learning style to ensure the effective attainment of desired skills. Encouraging Collaboration and Communication One of the ways educators can facilitate problem-solving is by promoting collaboration and communication among students. Working as a team allows students to share diverse perspectives while considering multiple solutions, thereby fostering an open-minded and inclusive environment that is crucial for effective problem-solving. Creating a Safe Space for Failure Educators must recognize that failure is an integral component of the learning process in a problem-solving method. By establishing a safe environment that allows students to fail without facing judgment or embarrassment, teachers enable students to develop perseverance, resilience, and an enhanced ability to learn from mistakes. Designing Relevant and Engaging Problems The selection and design of appropriate problems contribute significantly to the success of the problem-solving method of teaching. Educators should focus on presenting issues that are relevant, engaging, and age-appropriate, thereby sparking curiosity and interest amongst students, which further improves their problem-solving abilities. Scaffolding Learning Scaffolding is essential in the problem-solving method for providing adequate support when required. Teachers need to break down complex problems into smaller, manageable steps, and gradually remove support as students develop the necessary skills, thus promoting their self-reliance and independent thinking. Providing Constructive Feedback Constructive feedback from educators is invaluable in facilitating the problem-solving method of teaching, as it enables students to reflect on their progress, recognize areas for improvement, and actively develop their critical thinking and problem-solving abilities. In conclusion, the role of educators in facilitating the problem-solving method of teaching comprises promoting collaboration, creating a safe space for failure, designing relevant problems, scaffolding learning, and providing constructive feedback. By integrating these elements, educators can help students develop essential life-long skills and effectively navigate the complex world they will experience.
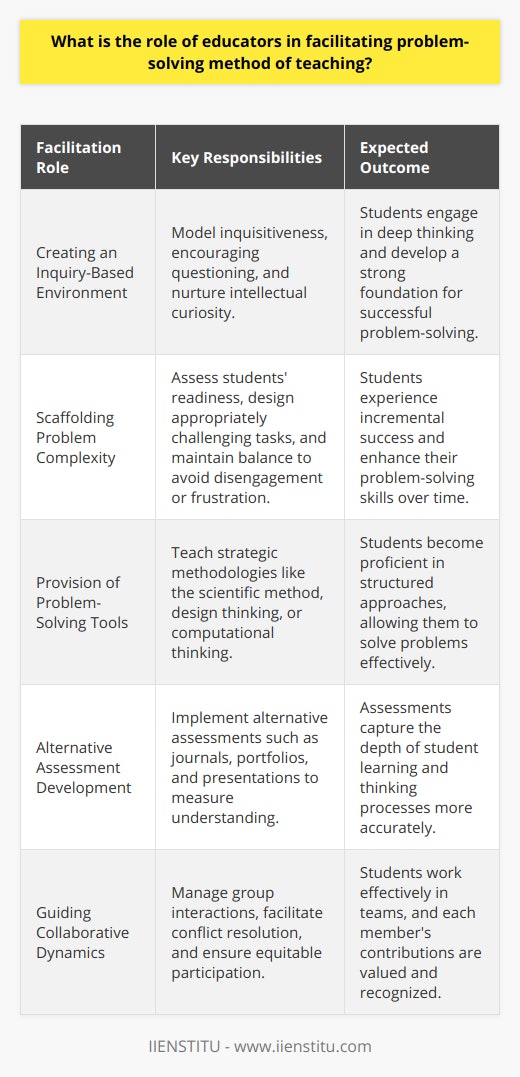
Can interdisciplinary approaches be incorporated into problem-solving teaching methods, and if so, how?
Interdisciplinary Approaches in Problem-Solving Teaching Methods Integration of Interdisciplinary Approaches Incorporating interdisciplinary approaches into problem-solving teaching methods can be achieved by integrating various subject areas when presenting complex problems that require students to draw from different fields of knowledge. By doing so, learners will develop a deeper understanding of the interconnectedness of various disciplines and improve their problem-solving skills. Project-Based Learning Activities Implementing project-based learning activities in the classroom allows students to work collaboratively on real-world problems. By involving learners in tasks that necessitate the integration of diverse subjects, they develop the ability to transfer skills acquired in one context to novel situations, thereby expanding their problem-solving abilities. Role of Teachers in Interdisciplinary Teaching Teachers play a crucial role in the successful incorporation of interdisciplinary methods in problem-solving teaching. They must be prepared to facilitate student-centered learning and engage in ongoing professional development tailored towards interdisciplinary education. In doing so, educators can create inclusive learning environments that encourage individualized discovery and the application of diverse perspectives to solve complex problems. Benefits of Interdisciplinary Teaching Methods Adopting interdisciplinary teaching methods in problem-solving education not only enhances students' problem-solving abilities but also fosters the development of critical thinking, creativity, and collaboration. These essential skills enable learners to navigate and adapt to an increasingly interconnected world and have been shown to contribute to students' academic and professional success. In conclusion, incorporating interdisciplinary approaches into problem-solving teaching methods can be achieved through the integration of various subject areas, implementing project-based learning activities, and the active role of teachers in interdisciplinary education. These methods benefit students by developing problem-solving skills, critical thinking, creativity, and collaboration, preparing them for future success in an interconnected world.
In what ways can technology be integrated into the problem-solving method of instruction?
**Role of Technology in Problem-Solving Instruction** Technology can be integrated into the problem-solving method of instruction by enhancing student engagement, promoting collaboration, and supporting personalized learning. **Enhancing Student Engagement** One way technology supports the problem-solving method is by increasing students' interest through interactive and dynamic tools. For instance, digital simulations and educational games can help students develop critical thinking and problem-solving skills in a fun, engaging manner. These tools provide real-world contexts and immediate feedback, allowing students to experiment, take risks, and learn from their mistakes. **Promoting Collaboration** Technology also promotes collaboration among students, as online platforms facilitate communication and cooperation. Utilizing tools like video conferencing and shared workspaces, students can collaborate on group projects, discuss ideas, and solve problems together. This collaborative approach fosters a sense of community, mutual support, and collective problem-solving. Moreover, it helps students develop essential interpersonal skills, such as teamwork and communication, which are crucial in today's workplaces. **Supporting Personalized Learning** Finally, technology can be used to provide personalized learning experiences tailored to individual learners' needs, interests, and abilities. With access to adaptive learning platforms or online resources, students can progress at their own pace, focus on areas where they need improvement, and explore topics that interest them. This kind of personalized approach allows instructors to identify areas where students struggle and offer targeted support, enhancing the problem-solving learning experience. In conclusion, integrating technology into the problem-solving method of instruction can improve the learning process in various ways. By fostering student engagement, promoting collaboration, and facilitating personalized learning experiences, technology can be employed as a valuable resource to develop students' problem-solving skills effectively.

I graduated from the Family and Consumption Sciences Department at Hacettepe University. I hold certificates in blogging and personnel management. I have a Master's degree in English and have lived in the US for three years.

What are Problem Solving Skills?

How To Solve The Problems? Practical Problem Solving Skills

A Problem Solving Method: Brainstorming

How To Develop Problem Solving Skills?
Wise-Answer
Find answers to all questions with us
What are the advantages of problem solving method of teaching?
Table of Contents
- 1 What are the advantages of problem solving method of teaching?
- 2 What are the cons of problem-based learning?
- 3 What is the advantage of problem-based learning?
- 4 Why is PBL bad?
- 5 Is problem-based learning effective?
- 6 What is problem solving in the classroom?
- 7 What happens when you teach students problem solving?
- 8 What are the advantages and disadvantages of problem solving?
Advantages of Problem-Based Learning
- 1. Development of Long-Term Knowledge Retention.
- Use of Diverse Instruction Types.
- Continuous Engagement.
- 4. Development of Transferable Skills.
- Improvement of Teamwork and Interpersonal Skills.
What are the cons of problem-based learning?
Risks of Problem-Based Learning
- Prior learning experiences do not prepare students well for PBL.
- PBL requires more time and takes away study time from other subjects.
- It creates some anxiety because learning is messier.
- Sometimes group dynamics issues compromise PBL effectiveness.
- Less content knowledge may be learned.
What are the pros and cons of having problem?
What are the pros and cons in having a problem?
- Promotion of deep learning.
- Developing rentation of knowledge in long term.
- Introduction to open-ended questions.
- Improved teamwork and interpersonal skills.
- Opportunity to apply skills in the real world.
What is the advantage of problem-based learning?
The key benefit of problem-based learning is that it develops students who are able to collaborate, solve problems, think clearly and connect prior knowledge to a problem.
Why is PBL bad?
Lack of a Real-World Connection Powerful PBL connects students with real-world learning around challenging questions. When PBL lacks the real-world connection, it can feel contrived and can lose the power to motivate students to engage in deeper learning.
Is being a good problem solver an advantage?
Good problem solving activities provide an entry point that allows all students to be working on the same problem. The open-ended nature of problem solving allows high achieving students to extend the ideas involved to challenge their greater knowledge and understanding. Problem solving develops mathematical power.
Is problem-based learning effective?
Students found PBLs as an effective strategy to promote teamwork and critical thinking skills. Conclusion: PBL is an effective method to improve critical thinking and problem solving skills among medical students.
What is problem solving in the classroom?
Problem-solving is the ability to identify and solve problems by applying appropriate skills systematically. Problem-solving is a process—an ongoing activity in which we take what we know to discover what we don’t know.
What are the pros and cons of problem based learning?
What happens when you teach students problem solving?
What are the advantages and disadvantages of problem solving.
Is there a right or wrong answer in problem based learning?
Share this post
Privacy overview.
| Cookie | Duration | Description |
|---|---|---|
| cookielawinfo-checkbox-analytics | 11 months | This cookie is set by GDPR Cookie Consent plugin. The cookie is used to store the user consent for the cookies in the category "Analytics". |
| cookielawinfo-checkbox-functional | 11 months | The cookie is set by GDPR cookie consent to record the user consent for the cookies in the category "Functional". |
| cookielawinfo-checkbox-necessary | 11 months | This cookie is set by GDPR Cookie Consent plugin. The cookies is used to store the user consent for the cookies in the category "Necessary". |
| cookielawinfo-checkbox-others | 11 months | This cookie is set by GDPR Cookie Consent plugin. The cookie is used to store the user consent for the cookies in the category "Other. |
| cookielawinfo-checkbox-performance | 11 months | This cookie is set by GDPR Cookie Consent plugin. The cookie is used to store the user consent for the cookies in the category "Performance". |
| viewed_cookie_policy | 11 months | The cookie is set by the GDPR Cookie Consent plugin and is used to store whether or not user has consented to the use of cookies. It does not store any personal data. |

IMAGES
COMMENTS
Advantages of Problem-Based Learning. 1. Development of Long-Term Knowledge Retention. Students who participate in problem-based learning activities can improve their abilities to retain and recall information, according to a literature review of studies about the pedagogy.. The literature review states "elaboration of knowledge at the time of learning" -- by sharing facts and ideas ...
The problem-solving method is an effective teaching strategy that promotes critical thinking, creativity, and collaboration. It provides students with real-world problems that require them to apply their knowledge and skills to find solutions. By using the problem-solving method, teachers can help their students develop the skills they need to ...
Nilson (2010) lists the following learning outcomes that are associated with PBL. A well-designed PBL project provides students with the opportunity to develop skills related to: Working in teams. Managing projects and holding leadership roles. Oral and written communication. Self-awareness and evaluation of group processes. Working independently.
Here's some of the information contained in the table. Benefits of Problem-Based Learning. For Students. It's a student-centered approach. Typically students find it more enjoyable and satisfying. It encourages greater understanding. Students with PBL experience rate their abilities higher. PBL develops lifelong learning skills.
Make students articulate their problem solving process . In a one-on-one tutoring session, ask the student to work his/her problem out loud. This slows down the thinking process, making it more accurate and allowing you to access understanding. When working with larger groups you can ask students to provide a written "two-column solution.".
Problem-based learning is a teaching method in which students' learn through the complex and open ended ... PBL is both a teaching method and approach to the curriculum. It can develop critical thinking skill, problem solving abilities, communication skills and lifelong learning. The purpose of this study is ... Some of the advantages are as ...
Problem-based learning (PBL) is a student-centered approach that teachers use. to promote students' critical thinking or analytical skills to solve real-life or. open-ended problems in a group ...
Problem-based learning (PBL) has been widely adopted in diverse fields and educational contexts to promote critical thinking and problem-solving in authentic learning situations. Its close affiliation with workplace collaboration and interdisciplinary learning contributed to its spread beyond the traditional realm of clinical education 1 to ...
Some common problem-solving strategies are: compute; simplify; use an equation; make a model, diagram, table, or chart; or work backwards. Choose the best strategy. Help students to choose the best strategy by reminding them again what they are required to find or calculate. Be patient.
structured problem solving. 7) Use inductive teaching strategies to encourage synthesis of mental models and for. moderately and ill-structured problem solving. 8) Within a problem exercise, help ...
Resolve Conflicts. In addition to increased social and emotional skills like self-efficacy and goal-setting, problem-solving skills teach students how to cooperate with others and work through disagreements and conflicts. Problem-solving promotes "thinking outside the box" and approaching a conflict by searching for different solutions.
In this way, the teaching content developed gradually as a group effort. Oral reports entailed group members introducing the definitions, connotations, type, and advantages/disadvantages of the teaching method. Finally, I integrated and summarised the main content with respect to the teaching instructions.
The problem-solving method of teaching is a student-centered approach to learning that focuses on developing students' problem-solving skills. In this method, students have to face real-world problems to solve. They are encouraged to use their knowledge and skills to provide solutions. The teacher acts as a facilitator, providing guidance and ...
Problem-solving is inherently student-centered. Student-centered learning refers to methods of teaching that recognize and cater to students' individual needs. Students learn at varying paces, have their own unique strengths, and even further, have their own interests and motivations - and a student-centered approach recognizes this ...
Given the effectiveness of the problem-solving method in improving students' learning and motivation, it should be used during physical education teaching. This could be achieved through the organization of comprehensive training programs, seminars, and workshops for teachers so to master and subsequently be able to use the problem-solving ...
Classroom instruction in problem solving often takes the form of presenting neat, verification-style problems to students at the end of a period of learning. This practice stands in stark contrast to professional problem solving, where the problem comes first, and is a catalyst for investigation and learning.
Problem-solving is the capacity to identify and describe a problem and generate solutions to fix it. Problem-solving involves other executive functioning behaviors as well, including attentional control, planning, and task initiation. Individuals might use time management, emotional control, or organization skills to solve problems as well.
Teaching about problem solving begins with suggested strategies to solve a problem. For example, "draw a picture," "make a table," etc. You may see posters in teachers' classrooms of the "Problem Solving Method" such as: 1) Read the problem, 2) Devise a plan, 3) Solve the problem, and 4) Check your work. There is little or no ...
Effectiveness ofProblem Solving Method in Teaching Mathematics at Elementary Level 234 According to Nafees (2011), problem solving is a process to solve problems ... In using the problem solving method, the subject matter must be organized on a basis of problem. The teacher must always be conscious of the practical value
The problem-solving method of teaching is the learning method that allows children to learn by doing. This is because they are given examples and real-world situations so that the theory behind it can be understood better, as well as practice with each new concept or skill taught on top of what was previously learned in class before moving onto another topic at hand.
The advantages and disadvantages of problem-solving practice when learning basic addition facts. In H. Askell-Williams & J. Orrell (Eds.), Problem solving for teaching and learning: A festschrift for emeritus professor Mike Lawson (pp. 209-227). ... Microgenetic methods are particularly valuable in illustrating this complex process and ...
Problem solving is not only an instructional goal, but also an instructional method. As an instructional method it can be used to build new mathematical knowledge, to solve problems that arise in ...
Advantages of Problem-Based Learning. 1. Development of Long-Term Knowledge Retention. Use of Diverse Instruction Types. Continuous Engagement. 4. Development of Transferable Skills. Improvement of Teamwork and Interpersonal Skills.